What is the speed of block bottom of encounters a loop

Question

Assignment:

Problems:

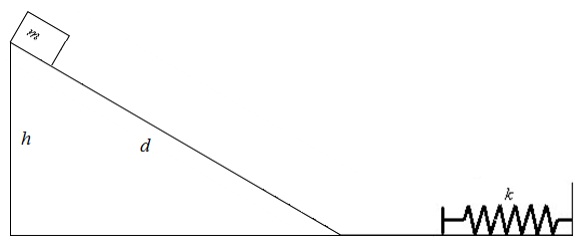
1. Mass m = 20kg is released from rest from the top of a frictionless ramp of height h = 1.8 m and length d = 2.4 m. At the bottom of the ramp, the mass slides on a horizontal, frictionless surface until it compresses a spring of spring constant k = 10 N/m.

a. What is the speed of the mass at the bottom of the ramp?

b. How far does the spring compress?

c. How much work does gravity do on the mass when it slides down the ramp?

d. How much work does the spring do on the mass during the compression?

e. What is the total work done on the mass during the interval from the moment it is released to the moment the spring is fully compressed?

2. A small block of mass m = 3.0 kg begins at height h = 18 m above the ground. It slides down a frictionless surface and encounters a loop of radius R = 6 m, as shown in the figure.

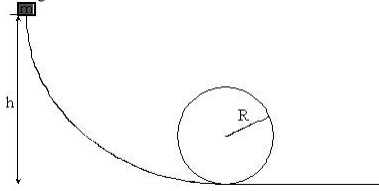
a. What is the speed of the block at the bottom of the loop?

b. What is the speed of the block at the top of the loop?

c. How much work does gravity do as the block moves from its highest to its lowest point?

d. How far will the block travel before stopping if a coefficient of kinetic friction μk = 0.25 exists between the block and the part of the track after the loop?

e. What is the work done by friction in this case?

3. Block 1 of mass m₁ = 5.0 kg slides from rest along a frictionless ramp from height h = 4.50 m and then collides and sticks with stationary block 2 of mass m₂ = 3.0 kg. After the collision, blocks 1 and 2 slide into a region where the coefficient of kinetic friction μk is 0.14 and comes to a stop in distance d within that region.

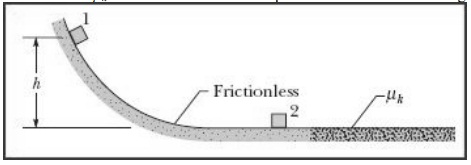
a. Calculate the speed of block 1 right before the collision.

b. Calculate the speed of blocks 1 and 2 after the collision.

c. What is the value of distance d?

d. How much kinetic energy is lost in the collision?

4. A bullet of mass m = 0.28 kg is fired into a block of mass M = 1.9 kg hanging from a massless rope of length L = 2.0 m. The block is initially a height y₁ = 0.45 m from the ground, and after the bullet sticks to the block, they both rise to a height y₂ = 1.5 m.

a. Calculate the speed of the block and bullet right after the collision.

b. Calculate the speed of the bullet before the collision.

c. How much mechanical energy is lost in the collision?

d. What type of collision is this? Explain.

5. Two objects of the same mass, m = 1.00 kg, are projected towards each other, as shown below. The first object initially has velocity (20.0m/s)iˆ. The second object has initial velocity (-10.0m/s) iˆ. After the glancing collision, the first object goes off with angle 2.00° above the positive x-direction and the second object goes off with angle 5.00° below the negative x-direction.

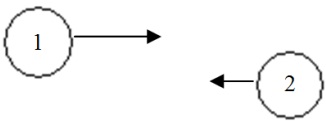
a. What is the speed of object 1 after the collision?

b. What is the speed of object 2 after the collision?

c. What is the change in kinetic energy in the collision?

d. Is this collision elastic or inelastic? Explain.