Discussion:
An airline company knows that over the long run, only 90% of passengers who reserve seats show up for their flight. On a flight with 300 seats, the airline accepts 324 reservations. Assume the passengers show up independently.
Q1. Give the upper bound on the probability that the flight will be overbooked (more than 300 people show-up).
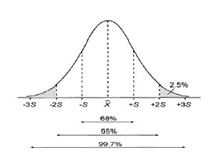
Q2. (Assume that the distribution of weight of US people is normal, with mean=70Kg and standard deviation 10Kg. Also, assume the plane is full. What is the probability that the overall weight of passengers exceeds 21346.4 Kg?
Q3. Again assuming the plane is full, the probability of each asking N cups of water is uniform in N:[1,2,3,4,5,6]. (Nobody asks 0 or more than 6 cups of water) How many cups of water will they need to load, in order to not run out of water in 99.85% of their flights?