Assignment:
Problems:
1) Derive the equation for the moment of inertia of a one dimensional stick of mass M and length L about an axis perpendicular to the stick through one end.
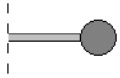
2) What is the moment of inertia about the y-axis for the system as shown. The stick has a length of 2L and a mass of M. The solid sphere has a diameter of L and a mass of 2M.
3) A merry-go-round of mass 150 kg and radius 1.5 m is set in motion by a rope that has been wrapped around it. Calculate the constant force that you would have to apply to the rope in order for the merry-go-round to start from rest and achieve and angular velocity of 0.5 rev/s in 2 s.
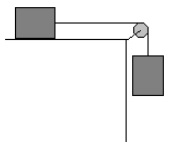
4) A mass of 8 kg is resting on a horizontal table with a coefficient of kinetic friction of 0.35. The mass is connected to a cable that passes
over a pulley of mass 3.5 kg and radius 21 cm. The other end of the cable is attached to a second hanging mass of 15 kg. The system is released from rest. (a) Calculate the acceleration of the system. (b) How many times does the pulley rotate in 2 s?
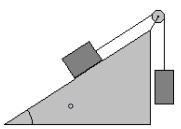
5) A mass of 5 kg is resting on a frictionless ramp. It is connected to a cable that passes over a pulley of radius 2 cm which is then attached to a second hanging mass. The system is moving at a constant velocity.
(a) Calculate the mass of the hanging block.
(b) If the blocks move 1.5 m in 3 seconds what is the angular velocity of the pulley?
6) A plank of wood 2 m long and mass 30 kg is supported by three ropes. A box of 700 N is resting on the plank. See diagram. Find the tension in each rope.
7) A beam has three forces acting on it as shown below. Sum the torques around (a) Point A and (b) Point B to find the net torque.

8) Mitch of 700 N walks out along a beam that is 6 m long and weighs 200 N. The box at the end weighs 80 N.
(a) Draw a Free-Body diagram for the beam.
(b) When Mitch is at x = 1 m, calculate the tension in the wire and the components of the reaction force at the hinge.
(c) The wire can handle a maximum tension of 900 N. How far out can Mitch walk before the wire will break?