Question 1.
Two (fictional) heavy particles are fired towards each other in a linear accelerator. Particle 1, moving towards the right, has a rest mass m1 = 16mp (mp is the proton mass) and velocity v1 = 3c/5 in the laboratory frame. Particle 2, moving towards the left, has a rest mass m2 = 9mp. The particles collide elastically and form a metastable particle, stationary in the laboratory frame.
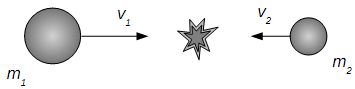
(a) Show, using conservation of total momentum before and after the collision, that the velocity of particle 2 in the laboratory frame is given by v2 = 4c/5.
(b) What is the mass of the metastable particle after the collision, in units of proton mass? Is mass conserved in the collision?
Now consider a reference frame co-moving with particle 1.
(c) In this reference frame, what is the velocity of particle 2?
(d) The rest mass is Lorentz invariant, and therefore independent of the choice of reference frame. Use conservation of energy and momentum in this new reference frame to prove that the collision will result in a particle of the same mass given by your answer to part (b) above, and moving to the left with velocity v1.
After some period of time, the metastable particle decays into a shower of lighter particles, including protons, electrons, neutrinos and photons.
(e) According to quantum mechanics, the shower of particles will scatter in all directions with equal probability. Is it possible to determine in advance the trajectory of any individual particle? Why?
(f) Several of the particles pass through a double-slit positioned near the point of the collision. Describe what happens as individual particles pass through it.
(g) Briefly describe how the resulting interference pattern will differ for the various types of particles passing through: protons, electrons, neutrinos (mass close to zero) and photons. Assume that the distribution of particle energies is the same for each kind of particle.
Question 2.
Recently, a new element has been isolated, temporarily named Ununseptium, with atomic number 117. The longest-lived isotope obtained so far is 294Uus with an atomic mass of 294:2105� 0:0007u.
(a) How many electrons does a neutral atom of 294Uus contain?
(b) What is the mass ms of one 294Uus atom, in SI units? Include the error estimate in your answer, using 1 atomic mass unit 1u = (1:66053892�0:00000007)�10 27 kg.
Suppose that one 294Uus atom is confined within a particle detector, a one-dimensional box of length L = 200 pm.
(c) What is the lowest possible kinetic energy of the atom in the box ? (3 significant figures is sufficient)
(d) If you measured the velocity of the atom while it is in the detector, what answer would you get?
(e) What velocity does the atom have in the absence of such a measurement?
(f) What is the total energy Es of the 294Uus atom?
294Uus is unstable, and decays rapidly into other atomic species. One possible decay route is via emission of an a-particle (a Helium nucleus, mass ma = 4:0001u) to form element 115 (Uup), with atomic mass mp = 290:1959u. Assume that the initial kinetic energy of the 294Uus atom can be neglected, so that the atom is approximately stationary in the lab frame.
(g) The total mass of the decay products is less than the mass of the original 294Uus atom. What accounts for this "missing mass" ?
(h) Conservation of total energy before and after the collision implies
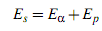
where Ea is the energy of the a particle, and Ep is the energy of the Uup atom.
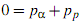
Similarly, conservation of total momentum before and after the collision implies Using these formulas, prove that the final energy of the a-particle is given by
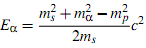
Hint: Solve for Ep, and use the mass-energy relation 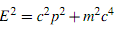
(i) Hence calculate the energy and velocity of the a-particle.