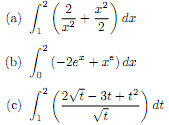What is the light intensity at the surface of the lake on

Question

Question 1.

As light passes through transparent materials (water, glass, plastic) some of the light is absorbed. The Beer-Lambert Law is used to describe the change in light intensity when light travels in such a material:

I = I₀e^-kx

where I₀ and I give the light intensity (in lumens) before and after passing though a distance x (in meter) of the material. The constant k depends on the type of material.

A researcher measures light intensity at dierent depths in a lake. At a depth of 2 m the light intensity is 12 lm, and at a depth of 3 m it is 7.9 lm.

(a) Use the experimental values to find the constant k for the water in the lake.
(b) What is the light intensity at the surface of the lake on the day of the experiment?
(c) At what depth will the light intensity be half of the intensity at the surface?
(d) If light intensity drops below 0.15 lm, a certain species of algae cannot survive. Find the depth below which there is insucient light for the algae to survive.

Question 2.

The standard form of the equation of a parabola is y = ax²+bx+c. In the figure the graphs of two parabolas are given.

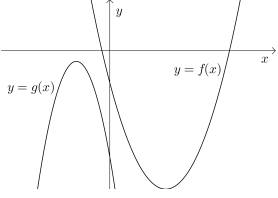
(a) For the two parabolas y = f(x) and y = g(x), are the coecients a, b and c positive or negative? Give reasons for your answers.

(b) In each case is b² > 4ac or b² < 4ac? Give reasons for your answers.

(c) For each case, supply a set of coecients that will produce a parabola similar to the given one.

Question 3. 

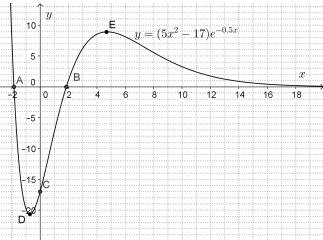
There are five points, labeled A to E, on the graph for the function f(x) = (5x² - 17)e-^0:5x. Find the coordinates for these points to 2 decimal digits, by using derivatives and algebra. Show your working.

Question 4.

(a) Use the algebraic definition of the derivative f'(x) = lim_h->0 {f(x + h) - f(x)}/h for find the derivative for the function f(x) = 1/2x + 5.
(b) Use the quotient rule to find the derivative of f(x) = 1/2x + 5. Show that your answer is the same as in (a).

(c) Use the algebraic definition as well as the quotient rule to find the derivative for f(x) = x/2-3x and again show that the answers are the same.

Question 5.

The relative growth rate of the world population has been decreasing steadily in recent years. Some population models predict that the world population will eventually stabilize at a level that the planet can support.

One such model (with t = 0 in 2000), is

P(t) = 73.2/6.1 + 5.9e^-0.02t

The relative growth rate of the population at time t is defined as P (t)/P(t).

(a) According to this model, what was the relative growth rate of the world population at the beginning of 2000? Give your answer as a percentage and round to 3 decimal digits.

(b) How does this value compare with the known relative growth rate of the world population in 2000? Give references for the data source(s) that you use.

(c) According to the model, what is the predicted relative growth rate for 2020?

Question 6.

The concentration of a certain medication in the bloodstream, t hours after taking it, is given by C(t) = 38t/t² + 2

(C in ppm, parts per million).

(a) Sketch a graph for the concentration.

(b) Calculate C0

(0:5) and C0

(5). Give the units for these rates.

(c) Explain the dierence in these rates by referring to the graph.

Question 7.

Sketch neat graphs of the curves y = 3 + 2x x2 and x = 3 y on the same coordinate system. Shade the region enclosed by the two curves. Use a definite integral to determine the area of this region. (You may use the online plotter on AUTonline for this question.)

Question 8.

Determine the following definite integrals.