Discuss the below:
Q1. For a "perfect" spring, the force applied to the spring as a function of amount of stretch is shown below.
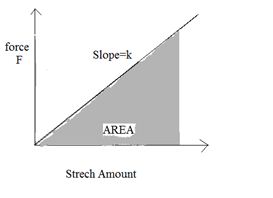
The work done is the area under the curve. Show that for an amount of stretch x, the work done is:
W =1/2kx^2
The easiest way is to use the diagram and the relation F = kx. (Note: you have seen the equation F = -kx. This is the force exerted by the spring. But to find the work done on the spring (which gives the spring its potential energy), we need the force exerted on the spring. This is opposite the force exerted by the spring.)
Q2. Spider silk is extremely strong and can be stretched as much as 30% before breaking. (Compare this with 2% for a steel wire.) Suppose that when a 50 mg spider is hanging by his thread, the silk thread is 34.9 mm long. With no force applied, the same thread would be 30.0 mm long. (We have used published figures for spider silk strength, to determine this amount of stretch.)
(A) Find the force constant k for that thread, treating it as a spring. Keep two significant figures.
(B) Suppose that something (like an air disturbance) causes the spider to begin oscillating up and down, like a mass on a spring. Find the frequency of the oscillation, in Hz.
Q3. Show that a simple pendulum 1.0 meter long has a period of pretty close to 2.0 seconds. This is sometimes useful for measurements of time if one does not have a watch, since the bob's motion from one side to the other takes very close to 1.0 second.
Q4. Suppose a pendulum has a period of 4.00 s when swinging on the Earth. (Assume g = 9.80 m/s2.) What would be the period of the same pendulum if placed on the moon, where g = 1.62 m/s2? (Note: you do not really needto find the length of the pendulum. See if you can do this problem without that.)
Q5. Suppose a 70-kg person has been climbing a "climbing wall" in an athletic facility, and is hanging from a 15-m length of nylon rope. (She has let go of the supports on the wall.) The effective spring constant of the rope is 1.5×104 N/m. The climber is oscillating up and down with amplitude of 2.0 cm.
(A) What is the frequency of oscillation?
(B) What is the total energy of the climber/rope "system"? (We are only considering the energy of oscillation, without worrying about gravitational potential energy due to the climber's height above the floor.)
(C) It is not possible for the climber in this situation to oscillate with amplitude of 5.0 cm, with simple harmonic motion. Show that this is a true statement. (Hint: There are at least two ways to do this. How much does the rope stretch when at equilibrium? What is the acceleration of the climber when she is at her maximum height in the cycle?)