What is the direction of the electric field at point p and

Question

1. In the figure below, q₁ = -2e, q₂ = +2e, q₃ = +4e, and q₄ = +2e, where e is the elementary charge. Charge q4 is located at the origin, while q₂ and q₃ are each a distance d₂ = d₃ = (2d)/3 from the origin. Charge 1 is a distance d₁ = d from the origin. The dashed line connecting charge 1 and charge 4 makes an angle θ₁ with respect to the negative x direction. Our goal is to find the net electric force on charge q₄.

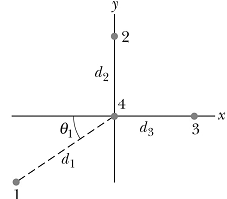
a. Let F₄₁ be the force that q₁ exerts on q4, F₄₂ be the force that q₂ exerts on q₄, and F₄₃ be the force that q3 exerts on q4. Draw vectors on the figure above that indicate the direction of each of these three forces (i.e. draw a free body diagram).

Remember to label each vector.

b. Determine
F₄₁ ,
and F₄₂, F₄₃, in unit vector notation. Put your answers in terms of d, θ1, and physical constants.

c. Determine the net force exerted on q4 in unit vector notation. Put your answer in terms of d, θ₁, and physical constants.
d. Determine the magnitude of F₄. Put your answer in terms of d, θ₁, and physical constants.

2. In the figure below, a non-conducting rod has a length L and a charge -q uniformly distributed along its length. Point P is a distance a from the right edge of the rod. Our goal in this problem is to find the electric field (both magnitude and direction) at point P. Assume that positive x is to the right.

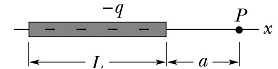
a. What is the direction of the electric field at point P?

b. Determine the linear charge density. Put your answer in terms of q, L, a, and physical constants.

c. Determine the electric field  due to a differential element of width dx, that is located a distance x from the left side of the rod. In other words, x = 0 at the left side of the rod. Put your answer in terms of q, L, a, x, dx, and physical constants.

d. Integrate to determine the net electric field at point P due to the entire rod. Put your answer in terms of q, L, a, and physical constants.

e. What is the electric field at point P if a is much larger than L? Let a → ∞ and determine the electric field using your answer from part d. Put your answer in terms of q, a, and physical constants. What type of charge distribution does the rod behave like when a is much larger than L?