Discuss the below in detail:
Q1: A sound source sends a sinusoidal sound wave of angular frequency 3000 rad / s and amplitude 12.0 nm through a tube of air. The internal radius of the tube is 2.00 cm. (a) What is the average rate at which mechanical energy is transported to the opposite end of the tube? If an identical wave and the original wave travel along the tube simultaneously, what is the total average rate at which they transport mechanical energy to the opposite end of the tube when their phase difference is (b) 0, and (c) 0.40 π rad?
Q2: A cylinder with a piston contains 0.250 mol of oxygen at 2.40105 Pa and 355 K. The oxygen may be treated as an ideal gas. The gas first expands isobarically to twice its original volume. It is then compressed isothermally to its original volume, and then it is cooled isochorically to its original pressure. (a) Show the series of processes on a pV - diagram. (b) Compute the temperature during the isothermal compression. (c) Compute the maximum pressure. (d) Compute the total work done by the piston on the gas. (e) Find the absolute value of the total heat flow into or out of the gas for this sequence of processes, and state the direction of heat flow.
Q3: A sinusoidal wave is traveling on a string with speed 40 cm / s. The displacement of the particles of the string at x = 10 cm is found to vary with time according to the equation y = (5.0cm) sin [1.0 - (4.0 s-1)t]. The linear mass density of the string is 4.0 g / cm. What are (a) the frequency and (b) the wavelength of the wave? If the wave function is of the form y(x, t) = A sin (kx ωt), what are (c) A, (d) k, (e) ω, and (f) the direction of the wave? (g) What is the tension in the string?
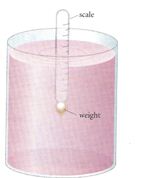
Q4: THE PHYSICS OF WINE: Dr. N. D. Tyson, an internationally known astrophysicist, is also a connoisseur of fine wines. According to a May 2000 Wine Spectator article, Dr. Tyson "...delves into the mysteries of classic Bordeaux with as much zeal as he brings to his exploration of the universe." At an after-hour session of a recent NSBP conference, ND presented a brief lecture on the art of wine making. He described several types of wine classifications for his audience; however his focus was a classification based on the wine's starting sugar content. To measure the sugar content, the alcoholic beverage industry employs the Brix scale. The calibration of this scale is based on the weight percent of sugar in solution. Dry wines have lower sugar content and thus lower specific gravity, whereas the specific gravity of sweet wines is higher. Ordinarily, a hydrometer is used to determine the specific gravity of a wine. Being a bigger fan of physics than of chemistry, Dr. Tyson argued that one could determine the specific gravity of a wine in the absence of a hydrometer by devising a physics experiment instead. To demonstrate this, he constructed a 100 g weighted cylinder of length L and cross-sectional area A by placing a weight at the cylinder's lower end. Thus the cylinder floated vertically when it was placed in a glass of wine. A length L* was submerged when the cylinder reached its equilibrium position (See the figure below.) Dr. Tyson then lifted the cylinder from its equilibrium position by an amount Δy, released it and timed its cycles. (Note: Δy is much less than the length of the cylinder.) (a) Show that the cylinder executes simple harmonic motion when it is released. That is, show that the restoring force is proportional to the cylinder's displacement from its equilibrium position. All work must be shown. List all physics principles used and all assumptions made. (b) In his first demonstration, Dr. Tyson measured a frequency of 1.195Hz. When he repeated this procedure for a second wine, the measured frequency was 1.160 Hz. Assume that Dr. Tyson lifted the cylinder 2.3 cm from its equilibrium position and that the cylinder's radius is 1.26 cm. Describe the displacement of the cylinder as a function of time as it oscillates in the dry wine with an equation of the form y (t) = A sin (ωt + ?). Your expression must include values for the amplitude A, the angular frequency ω, and the phase constant ? of this body. (c) Find the time at which the speed of the cylinder is - 0.112 m / s. (d) What is the value of the acceleration at this time? (e) Calculate the density of the dry wine.