Assignment:
Problem 1: Rigid Body & Rotational Dynamics: Moment of Inertia & Torque
A wheel free to rotate about its axis that is not frictionless is initially at rest. A constant external torque of 36 N-m is applied to the wheel for 22 sec, giving the wheel an angular velocity of +4200 rev/ min. The external torque is then removed, and the wheel comes to rest 220 sec later.

a) Find the moment of inertia of the wheel.
b) Find the frictional torque, which is assumed to be constant.
Problem 2: Conservation of Momentum: Multi-dimensional Collision Remember that momentum is a vector. Consider an example with a projectile (m1) hitting a target (m2) initially at rest following a glancing collision (not head on) as shown below.

(a) Is the momentum conserved in this type of collision? If yes, write down the appropriate equations to support your claim.
(b) Assuming energy is conserved in this collision; express the related energy conservation equation?
(c) Assume the type of collision is uncertain. If mass (m2) equals to the twice the mass of m1, and the recoiled angles are given as θ1 (60o
) for m1, and θ2 (30o ) for m2 respectively after the collision, derive all possible corelations among all components of velocities (V1,i; V1,f; V2,f) in sequence starting from part (a). Determine whether the collision is elastic or in-elastic type in nature with your logistic evidence.
[Given: Sin30 o = Cos60o = 1/2; and Cos30o = Sin60o = √3/2]
Problem 3: Mechanical Energy&Work done by Conservative/Non-conservative Forces
A block of mass m = 1 Kg sits on a θ = 45° slope and is held in place by a spring of natural length L0 = 10 cm and spring constant ks = 282 N/m. Use g = 10 m/s2 and √2≈ 1.41.
a. If there's no friction between the block and the slope, what is the length L of the spring when it holds the block at rest? Express L as a function of L0, m, ks and θ before plugging in any numbers.
b. If there is a static friction coefficient μS = 0.5 between the block and slope, what are the maximum and minimum lengths Lmax and Lmin between which the block may be at rest? Express Lmax and Lmin as a function of L0, m, kS, μS and θ before plugging in any numbers. It's very important to draw the block's FBD at the maximum and minimum positions!
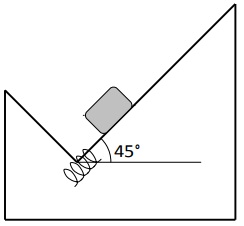
Problem 4: Work done and Kinetic Energy Theorem
A mass m = 8 kg, is attached to a spring, with spring constant k = 50 N/m and sits at equilibrium state on a horizontal surface. The region of the surface to the right of the equilibrium point is frictionless, whereas the region to the left of the equilibrium has friction, with µk = 0.01. Take the equilibrium point to be xo = 0. Use g=10 m/s2
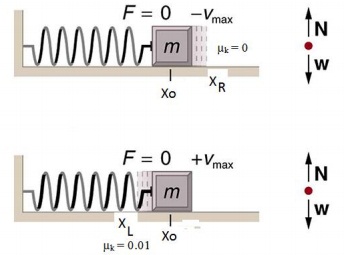
Derive formulas before plugging in any numbers.
a) The mass is moved to a position xR= 0.2 m, to the right of the equilibrium point (x0). Explicitly calculate (ie: starting from the integral definition) the work done by the spring as the mass is moved from the equilibrium position to xR.
b) The mass is then released at xR and allowed to return to its equilibrium position (x0). How fast (Vmax) is the mass moving when it returns to its equilibrium assuming its motion to be perfectly harmonic.
c) The mass passes through its equilibrium position, and continues moving to the left over the segment of the surface with friction. What is the maximum position, xL, reached by the mass?
Problem 5: Work -Kinetic Energy Theorem
Toy car on top of the hoop: A toy car of mass m = 0.100 kg is launched on a horizontal track by a spring (constant kS = 2500 N/m) compressed by a distance d = 2.00 cm. The track curves vertically into a circular loop of radius (R) = 10.0 cm. All friction can be neglected and you should use g = 10 m/s2 .
What is the normal force A (magnitude and direction) felt by the car at point A (highest point in loop)?
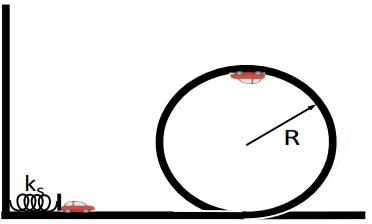
(1) Draw the FBD for the car at point A (highest point in loop);
(2) Express the result as function of m, g, ks, d, and R before plugging in any values;
(3) Ensure units and significant figures are consistent! Use g=10.0 m/s2.
Problem 6: Rotational Kinematics-Moment of Inertia: Ceiling Fan & Spinning Disk
Case A: Ceiling Fan
The next nine questions pertain to the situation described below
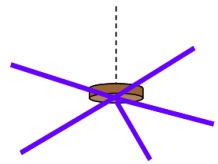
A ceiling fan consists of a small cylindrical disk with 5 thin rods coming from the center. The disk has mass md = 3.3 kg and radius R = 0.22 m. The rods each have mass mr = 1.2 kg and length L = 0.78 m.
1) What is the moment of inertia of each rod about the axis of rotation?
2) What is the moment of inertia of the disk about the axis of rotation?
3) What is the moment of inertia of the whole ceiling fan?
4) When the fan is turned on, it takes t = 3.5 s and a total of 16 revolutions to accelerate up to its full speed. What is the magnitude of the angular acceleration?
5) What is the final angular speed of the fan?
6) What is the final rotational energy of the fan?
7) Now the fan is turned to a lower setting where it ends with half of its rotational energy as before. The time it takes to slow to this new speed is also t = 3.5 s. What is the final angular speed of the fan?
8) What is the magnitude of the angular acceleration while the fan slows down?'
Case B: Spinning Disk
The next nine questions pertain to the situation described below.
A disk with mass m = 11.5 kg and radius R = 0.3 m begins at rest and accelerates uniformly for t = 16.6 s, to a final angular speed of ω = 29 rad/s.
9) What is the angular acceleration of the disk?
10) What is the moment of inertia of the disk about the axis of rotation?
11) What is the angular displacement over the 16.6 s?
12) What is the change in rotational energy of the disk?
13) What is the tangential component of the acceleration of a point on the rim of the disk when the disk has accelerated to half its final angular speed?
14) What is the magnitude of the radial component of the acceleration of a point on the rim of the disk when the disk has accelerated to half its final angular speed?
15) What is the final speed of a point on the disk half-way between the center of the disk and the rim?
16) What is the total distance a point on the rim of the disk travels during the 16.6 seconds?
Poblem 7: Rotational Kinematics-Moment of Inertia: Sliding Sphere-Hanging Hoop
Case A: Sliding and Rolling Sphere: Bowling Ball
A spherical bowling ball with mass m = 4.2 kg and radius R = 0.101 m is thrown down the lane with an initial speed of v = 8.2 m/s. The coefficient of kinetic friction between the sliding ball and the ground is μ = 0.29. Once the ball begins to roll without slipping it moves with a constant velocity down the lane.
(a) What is the magnitude of the angular acceleration of the bowling ball as it slides down the lane?
(b) What is magnitude of the linear acceleration of the bowling ball as it slides down the lane?
(c) How long does it take the bowling ball to begin rolling without slipping?
(d) How far does the bowling ball slide before it begins to roll without slipping?
(e) What is the magnitude of the final velocity?
(f) After the bowling ball begins to roll without slipping, compare the rotational and translational kinetic energy of the bowling ball:
Case B: Sphere with hanging hoop problem

An green hoop with mass mh = 2.8 kg and radius Rh = 0.12 m hangs from a string that goes over a blue solid disk pulley with mass md = 1.9 kg and radius Rd = 0.09 m. The other end of the string is attached to a massless axel through the center of an orange sphere on a flat horizontal surface that rolls without slipping and has mass ms = 3.8 kg and radius Rs= 0.22 m. The system is released from rest.
1) What is magnitude of the linear acceleration of the hoop?
2) What is magnitude of the linear acceleration of the sphere?
3) What is the magnitude of the angular acceleration of the disk pulley?
4) What is the magnitude of the angular acceleration of the sphere?
5) What is the tension in the string between the sphere and disk pulley?
6) What is the tension in the string between the hoop and disk pulley?
7) The green hoop falls a distance d = 1.69 m. (After being released from rest.)
How much time does the hoop take to fall 1.69 m?
8) What is the magnitude of the velocity of the green hoop after it has dropped 1.69 m?
9) What is the magnitude of the final angular speed of the orange sphere (after the green hoop has fallen the 1.69 m)?