What are the decision variables

Question

Problems:

1.  Consider the following maximum problem in standard form:

Maximize Z = 8X₁ + 2X₂ + 3X₃

subject to the constraints

  X₁ + 3X₂ + 2X₃ < 10

4X₁ + 2X₂ + 3X₃ <   8

   X₁ > 0,  X₂ > 0,  X₃ > 0

(a)  Rewrite the two constraints as equations by adding slack variables S₁ and S₂.

(b)  Set up the initial simplex tableau for this problem.

In problems 2 -4, each tableaux represents a step in the solution of a maximization problem in standard form.  Determine if the tableaux:

            (i)   is the final tableaux

            (ii)  requires additional pivoting

            (iii)  indicates no solution to the problem

If the answer is (i), write down the solution; if the answer is (ii), identify the pivot element.

2.

3. 

4. 

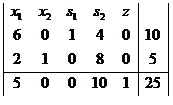
5.  A brewery manufactures three types of beer - lite, regular, and dark.  Each vat of lite beer requires 6 bags of barley, 1 bag of sugar and 1 bag of hops.  Each vat of regular beer requires 4 bags of barley, 3 bag of sugar and 1 bag of hops.  Each vat of dark beer requires 2 bags of barley, 2 bag of sugar and 4 bag of hops.  Each day the brewery has 800 bags of barley, 600 bag of sugar and 300 bag of hops.  The brewery realizes a profit of $10 per vat of lite beer, $20 per vat of regular beer, and $30 per vat of dark beer.  For this linear programming problem:

(a) What are the decision variables?

(b) What is the objective function?

(c) What are the constraints?

E.C.  Solve the linear program in problem #5.