1. For the beam shown below, derive the equation of motion in the x- and y-directions. Recall that the deformation of a bar along its axis is:

2. The single-degree-of-freedom model below is subject to the pulse loading shown.
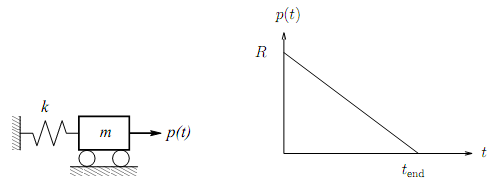
(a) If the mass starts at rest, verify that the displacement response of the system, x(t), during the pulse (0 ≤ t ≤ tend) is as shown below. Note that you can verify the solution if x(t) satisfies the initial conditions and the equation of motion.
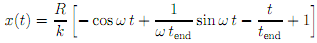
(b) Using the valuesR = 10 kip, tend = 1.0 sec,m= 0.100 kip sec2/inch, k = 10.0 kip/inch, find the equation for displacement, x(t), after the pulse stops (t > tend). Your solution does not need to be symbolic, you can give numeric values, just be sure all units are consistent.
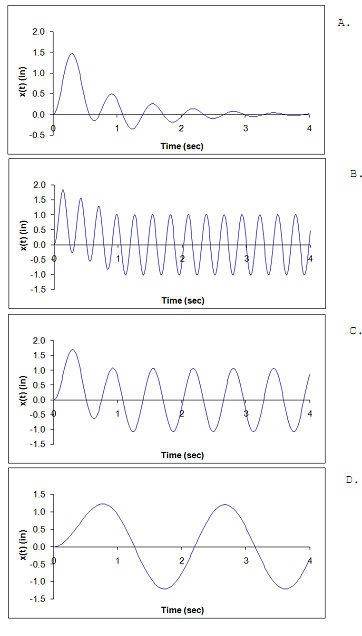
(c) Included with the exam are possible graphs for x(t). Determine which graph is correct. All parameters are the same as part (b). Explain your answer.
3. The 400,000 gallon water tower below has four 50-foot steel columns (H = 50 feet).
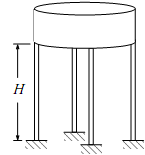
(a) When the tank is full it is observed that the natural period is approximately 1.8 seconds. When the tank is half full, the natural period is approximately 1.3 seconds. Using this information, find the approximate weight of the tank and the stiffness of the four columns. Use 8.3 lbs/gallon as the density of water and you may neglect the weight of the columns.
(b) Assuming that the columns are connected to the tank so that they do not rotate at the top, find the moment of inertia for the columns.