Assignment:
Question 1. A projectile of mass 0.6 kg is fired vertically upwards in the barrel of a gun by a vertical net propelling force of 50 N acting over a period of 0.5 seconds.
Calculate:
(a) The velocity of the projectile when it leaves the gun.
(b) The height reached by the projectile.
(c) The time taken from the projectile leaving the gun to its return to the same height.
Question 2. A flywheel (A) with a mass of 100 kg and radius of gyration 1200 mm rotates at 150 revs min–1 (clockwise). The kinetic energy of this flywheel is to be reduced by 20% by impacting it with a second flywheel (B) rotating at 80 revs min–1 in the opposite direction, such that they have the same (clockwise) angular velocity after impact.
(a) Calculate the required mass of flywheel (B) if its radius of gyration is 800 mm.
(b) Calculate the energy lost to the surroundings.
(c) Is the impact elastic? Give reasons for your answer.
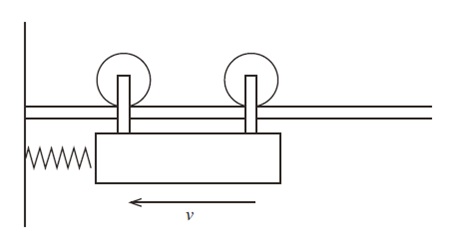
Question 3. A container of mass 100 kg runs on overhead rails. The container is mounted on two wheels each having a mass of 15 kg, a diameter of 1.2 m and a radius of gyration of 0.4 m.
Calculate:
(a) The total kinetic energy of the vehicle when travelling at 4 m s–1.
(b) The spring stiffness required to bring the vehicle to rest in a distance of 300 mm.
Question 4. For a given spring-mass system, what would be the effect on ω of:
(a) Increasing the size of the mass
(b) Increasing the amplitude of vibration
(c) Increasing the spring stiffness
(d) Increasing the phase lag.
Question 5. A mass attached to the lower end of a vertical spring causes the spring to extend by 25 mm to its equilibrium position. The mass is then displaced a further 20 mm and released. A trace of the vibration and time measurements are taken. From these measurements it can be seen that the displacement from the equilibrium position is 19.2 mm when the time is 0.05 s.
(a) Calculate the expected frequency of vibration.
(b) Calculate the maximum acceleration of the mass.
(c) Calculate the maximum velocity of the mass.
(d) Write the expression for the displacement of the mass as a function of time.
(e) Write the expression for the velocity of the mass as a function of time.
(f) Write the expression for the acceleration of the mass as a function of time.