Assignment:
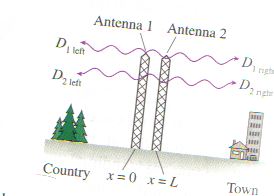
The broadcast antenna of an Am radio station is located at the edge of town. The station owners would like to beam all of the energy into town and none into the countryside, but a single antenna radiates energy equally in all directions. The attached figure shows two parallel antennas separated by a distance L. Both antennas broadcast a signal at wavelength lambda, but antenna 2 can delay its broadcast relative to antenna 1 by a time interval delta t in order to create a phase difference delta phi_0 between the sources. Your task is to find values of L and delta t such that the waves interfere constructively on the town side and destructively on the country side.
We are given this additional information:
Let antenna 1 be at x = 0. The wave that travels to the right is asin[2pi(x/lambda - t/T)]. The wave that travels to the left is asin[2pi(-x/lambda - t/T)]. Antenna 2 is at x = L. It broadcasts waves asin[2pi((x-L)/Lambda - t/T) + phi_20] to the right and asin[2pi(-(x-L)/lambda - t/T) + phi_20] to the left.
a) What is the smallest value of L for which you can create perfect constructive interference on the town side and perfect destructive interference on the fountry side? Your answer will be a multiple or fraction of the wavelength lambda.
b) What phase constant phi_20 of antenna 2 is needed?
c) What fraction of the oscillation period T must delta t be to produce the proper value of phi_20?
d) Evaluate both L and delta t for the realistic AM radio frequency of 1000 KHz.