Question 1) Consider the Solow Growth model without population growth or technological change. The parameters of the model are as described in the table below:
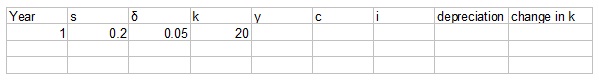
where s is the saving rate, δ is the depreciation rate, k is the capital stock per worker, y is output per worker, c is consumption per worker, and i is investment per worker.
a) Assume that our production function is given by Y = K1/3L2/3. Rewrite this production function in terms of output per worker as a function of capital per worker.
b) With the production function in a), fill out the first row of the table above.
c) Again using the production function from a), find the steady state level of capital in this economy.
d) Use Excel to fill out the above table for years 1-10. Then extend the table until the level of capital per worker is within 1 of the steady state value. In which year does k reach this point? (Note: you need only turn in the first 10 years of the table).
e) What is the Golden Rule level of k for this economy? Report your answer to two decimal places.
f) Assume that a benevolent social planner wishes to get this economy to the Golden Rule level of k. What savings rate must he impose on individuals to make kgold the steady state level of k? (Hint: this problem will be easier if you use the entire value of kgold from e), not the rounded value you reported.)
g) Assume that the economy begins at the steady state level of k you found in part c). Do you think the current citizens of this country support the planner’s decision to reach the Golden Rule level of k? Why or why not?
Question 2) In the country of Grand Fenwick, the steady state growth rate of real GDP is 8% per year. The population growth rate is 3%, while the rate of technological progress is 5%. The capital stock is 5 times as large as Grand Fenwick’s GDP, and 2% of the capital stock wears out each year. Capital income accounts for half of GDP.
a) Is Grand Fenwick at kgold?
b) If researchers in Grand Fenwick discover a way to double the rate of labor-augmenting technological progress, what will happen to kgold? What can we say about the growth rates of capital per effective worker and output per effective worker in the years following the breakthrough?
c) Comparing the steady states before and after the breakthrough, what happens to the growth rates of capital, output, and consumption per worker?
Question 3) The nation of Quodium has the production function Y = 20K1/4(EL)3/4 and a depreciation rate of 7%. The population of Quodium increases 4% each year, and the rate of technological progress is 5% per year. Assume that all residents of Quodium save 6.4% of their income each year.
a) Rewrite the production function in terms of output per effective worker (y) and capital per effective worker (k). Find the steady state levels of capital per effective worker, output per effective worker, consumption per effective worker, and investment per effective worker.
b) What is the steady state growth rate of GDP in Quodium? What is the steady state growth rate of GDP per worker?