Square cavity flow

Question

The figure shows a “square cavity flow”. In this flow the cavity is filled with a fluid and the lid of the cavity moves with a constant velocity, while the other walls stay stationary. This causes a recirculation in the cavity. The flow is supposed 2 dimensional incompressible, with U_max=1.0 m/s constant. The lengths of the sides of the cavity, L, are all equivalent to 1.0 m

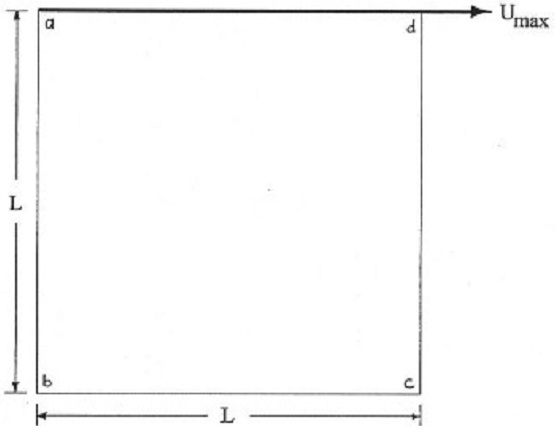
Run the program for the following Reynolds numbers: 10, 100, 500 and 1000. The Reynolds number is defined by Re = U_max.L/ν, where ν is the fluid kinematic viscosity, which in this case, differs to produce the dissimilar Reynolds numbers. The boundary conditions are: u_ab = u_bc = u_cd = v_ab= v_bc= v_cd = v_ad = 0 and u_ad = U_max

Employ a staggered grid of at least 15x15 cells, and the SIMPLE algorithm for your solutions.

Plot both velocity and pressure distributions for all Reynolds numbers.