Solving for temperatures of points on a flat square plate

Question

Problems:

Linear Algebra : Solving for Temperatures of Points on a Flat Square Plate

The concept of thermal resistance described in Problem 5 can be used to find the temperature distribution in the flat square plate shown

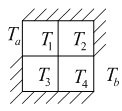
The plate's edges are insulated so that no heat can escape, except at two points where the edge temperature is heated to Ta and Tb, respectively. The temperature varies through the plate, so no single point can describe the plate's temperature. One way to estimate the temperature distribution is to imagine that the plate consists of four sub-squares and to compute the temperature in each sub-square. Let R be the thermal resistance of the material between the centers of adjacent sub-squares. Then we can think of the problem as a network of electrical resistors, as shown in Figure 5(b). Let qij be the heat flow rate between the points whose temperatures are Ti and Tj. If Ta and Tb remain

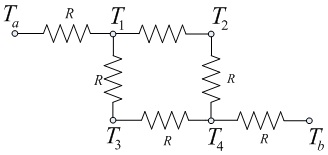
Constant for some time, then both the heat energy stored in each sub-square and the heat flow rate between each sub-square are constant. Under these conditions conservation of energy says that the heat flow into a sub-square equals the heat flow out. Applying this principle to each sub-square gives the following equations:

                                       q_a1 = q₁₂ + q₁₃                  q₁₂ = q₂₄       q₁₃ = q₃₄       q₃₄ + q₂₄ = q_4b

Substituting q_ij = Tⁱ - T^j/R , we find that R can be canceled out of every equation, and the equations can be rearranged as follows:

           T₁ = 1/3 (T_a + T₂ + T₃)                T₂ = 1/2 (T₁+T₄)             T₃ = 1/2 (T₁+T₄)              T₄ = 1/3 (T₂ + T₃ + T_b)

These equations tell us that the temperature of each sub-square is the average of the temperatures in the adjacent sub-squares

Solve these equations for the case where Ta = 150⁰C and Tb = 20⁰C