Question 1. For each of the following examples take the given information and write an equation in slope intercept form. Show all your work and NOT just your final answer.
a. The points (x, y) = (1, 7) and (200, -590) both sit on the same line.
b. The points (x, y) = (0, 20) and (2, 34) both sit on the same line.
c. The slope of the line is 5 and the line contains the point (x, y) = (3, 23).
d. The reciprocal of the slope for the line is 2 and the line contains the point (x, y) = (10, 1).
e. The point (x, y) = (5, 0) is on the line and furthermore you know that each time x increases by 5 units, y decreases by 10 units.
f. The point (x, y) = (10, 11) is on the line and furthermore you know that each time x increases by 4 units, y increases by 2 units.
Question 2. Find the solution (x, y) for each set of equations. Show your work and NOT just your final answer.
a. y = 20 - 2x
y = 12
b. y = 20 - 2x
x = 8
c. y = 20 - 2x
y = 40 + 2x
d. y = 20 - 2x
y = -20 + 2x
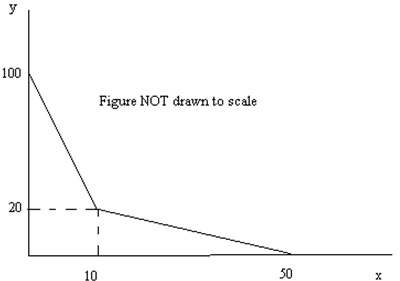
Question 3. You are provided the following figure and given another equation, y = (1/2)x + 2. Find the solution (x, y) given this information. (Hint: do NOT expect nice numbers! Do try to work this without a calculator though-it will be good practice.)
Question 4.
a. Suppose you are given a line described by the equation y = 50 - 4x and you are told that the x value has increased by 10 units at every y value. What is the equation for the new line? Show your work.
b. Suppose you are given a line described by the equation y = 50 - 4x and you are told that the x value has doubled at every y value. What is the equation for the new line? Show your work.
c. Suppose you are given a line described by the equation y = 50 - 4x and you are told that the y value has increased by 10 units at every x value. What is the equation for the new line? Show your work.
Question 5. Joe has $100 he plans to place in a savings account for the next 5 years. The bank will pay him 5% interest a year and Joe plans to keep his funds plus any interest earned in the account for the five years. How much money will Joe have in his account at the end of five years? Provide a formula for calculating the amount of money Joe would have in his account if he kept his money in the bank for 10 years? For twenty years? For thirty years?
Question 6. The Madison Dribblers, a recreational basketball team, has seven team members whose average height is 5 feet 10 inches. Suppose the team adds three new players whose heights are five foot six inches, six foot two inches, and six foot eight inches. What will be the new average height of the team? Show your work. (Hint: you may find it easiest to work in inches and then convert your final answer into feet and inches.)
Question 7. George and Mario both produce bicycles and sewing machines. George can produce one bicycle in ten hours and one sewing machine in five hours. Mario can produce one bicycle in eight hours and one sewing machine in six hours. Assume that the production possibility frontiers for George and Mario are linear and that they both have the same amount of time available to produce bicycles and sewing machines.
a. What is the slope of George's production possibility frontier if this PPF is measured with bicycles on the vertical axis and sewing machines on the horizontal axis?
b. What is the slope of Mario's production possibility frontier if this PPF is measured with bicycles on the vertical axis and sewing machines on the horizontal axis?
c. Given the above information, who has the comparative advantage in the production of bicycles?
d. Given the above information, who has the comparative advantage in the production of sewing machines?
e. Suppose that George and Mario each have 240 hours of labor they can use to produce either sewing machines or bicycles. Draw a production possibility frontier that represents their joint PPF given this information and the initial information.
f. What is the acceptable range of trading prices measured in sewing machines for 20 bicycles?
g. What is the acceptable range of trading prices measured in bicycles for 36 sewing machines?
Question 8. Yuri produces widgets and gadgets. If Yuri spends all of his time producing widgets he can produce 500 widgets and if he spends all of his time producing gadgets he can produce 350 gadgets. Yuri finds that for the first 100 gadgets he produces he must give up 1/2 widget for every gadget that he produces. Yuri finds for the second 100 gadgets he produces he must give up 1 widget for every gadget he produces. Yuri finds when he increases his production of gadgets from 200 gadgets to 300 gadgets he must give up 1.25 widgets for every gadget he produces. Finally Yuri knows that his PPF is linear between 300 gadgets and 350 gadgets.
a. Construct a graph illustrating Yuri's PPF. In your graph, measure widgets on the vertical axis and gadgets on the horizontal axis. Assume that Yuri's PPF is composed of a set of linear segments based upon the initial information you were given.
b. What is the opportunity cost of Yuri producing one additional gadget if he is currently producing 300 gadgets?