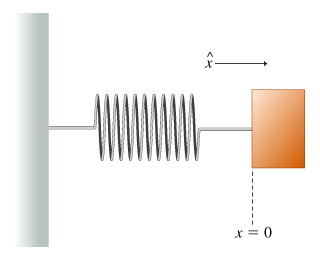
Assignment:
A block of mass m is attached to a spring whose spring constant is k. The other end of the spring is fixed so that when the spring is unstretched, the mass is located at x=0. Assume that the +x direction is to the right.
The mass is now pulled to the right a distance A beyond the equilibrium position and released, at time t=0, with zero initial velocity.
Assume that the vertical forces acting on the block balance each other and that the tension of the spring is, in effect, the only force affecting the motion of the block. Therefore, the system will undergo simple harmonic motion. For such a system, the equation of motion is a(t)= -k/m x(t),
and its solution, which provides the equation for x(t), is
x(t)= Acos(sqrt k/m t)
a). Find the velocity v of the block as a function of time.
Express your answer in terms of some or all of the variables: k, m, A, and t.
b). Find the kinetic energy K of the block as a function of time.
Express your answer in terms of some or all of the variables: k, m, A, and t.
c). Find K_max, the maximum kinetic energy of the block.
Express your answer in terms of some or all of the variables: k, m, and A.