Prove the parallellogram law for two complex numbers z and

Question

a) Prove the following "Parallellogram law'' for two complex numbers z and w:

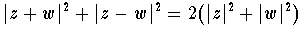
b) Let f(x) = x - Cos(x)

Show that the equation f(x) = x - cos(x)=0 has only one solution x₀ in the interval (Π/6, Π/4).

a) Derive the general solution of the identity:

(dy/dx + y)e^x = x.

a) Derive the Fourier Transform of the function:

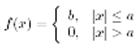
b) Using the result in Q4(a), plot the Fourier Transform graph

c) Apply the convolution theorem to functions:

f(x) = e-^αx2

g(x) = e^-bx2