Assignment:
Metallic alloys A, B, and C are to be made to customer specifications from four different metals (W, X, Y, and Z) that are extracted from two different ores. The cost, maximum available quantity, and constituent parts of these ores are: Customer specifications and selling price for the three alloys are:
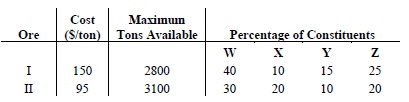
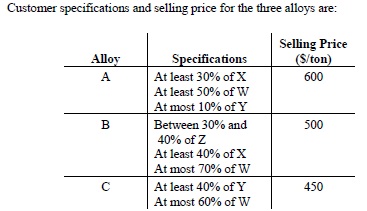
a) Formulate Linear Programming model for the problem to maximize the profit from the sale of the alloys. Clearly define all of your decision variables, objective and constraints
b) Solve the model using Excel and report the optimal solution. Include a snapshot of your solution.
c) If you can increase the availability of one of the ores, which one would you increase and why? Explain your answer based on the solution you obtained above without resolving the model.