Assignment:
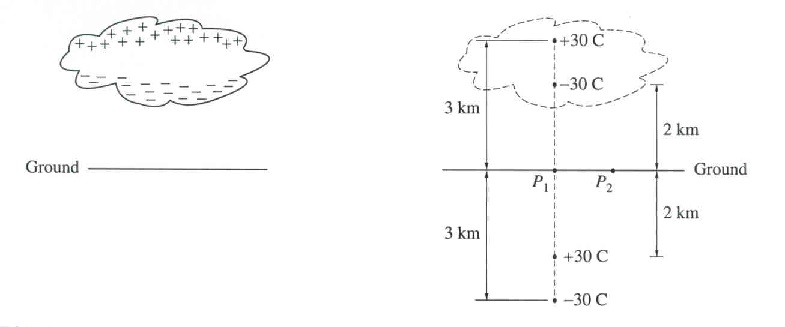
Question 1. A thundercloud has the charge distribution as shown on the diagram. Treat this distribution as two point charges, a negative charge of -30 C at the height of 2 km above the ground and a positive charge of +30 C at the height of 3 km. The presence of these charges induces charges on the ground. Assuming the ground is a conductor, it can be shown that the induced charges can be treated as a charge of +30 C at the depth of 2 km below ground level and a charge of -30 C at the depth of 3 km. Consider point P1, which is just above the ground directly below the thundercloud, and point P2, which is 1 km horizontally away from P1.
a) Determine direction and magnitude of electric field at these points.
b) Letting zero of potential to be at infinity, determine the potential at these points.
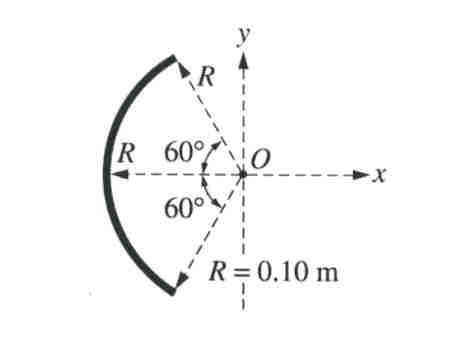
Question 2. A rod of uniform linear charge density +1.5 x 10-5 C/ m is bent into an arc of radius R = 0.10 m. The arc is placed with its center at the origin of the axis. A proton is placed at point O and held at rest. a) Determine the magnitude and direction of the force required to keep the proton at rest. (Ignore the effects of gravity) .b) Determine the electric potential at point O.
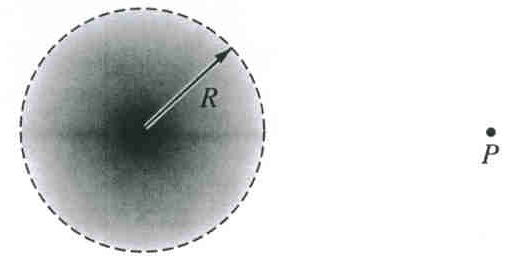
Question 3. A spherical cloud of radius R contains total charge +Q with a nonuniform volume charge density that varies according to the equation for and for , where r is the distance from the center of the cloud. Determine electric field E and electric potential U as a function of r . If proton is placed in point P and released, what is it going to move like after a long time after its release?
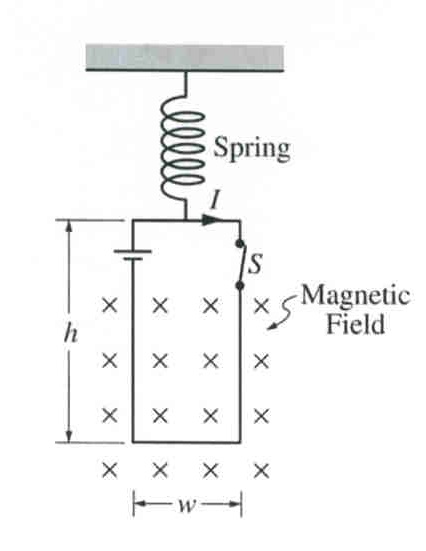
Question 4. A loop of wire of width w and height h contains a switch and a battery and is connected to a spring of force constant k. The loop carries a current I in a clockwise direction, and its bottom is in constant, uniform magnetic field directed into the plane of the page. a) If the switch is opened, the loop eventually comes to rest at a new equilibrium position that is a distance x from its former position. Derive an expression for the magnitude B of the uniform magnetic field in terms of given quantities and fundamental constants. b) The loop is replaced by the loop of the same dimensions and resistance R but without the battery and switch. This loop is pulled upward, out of magnetic field, at constant speed v. Derive an expression for the magnitude of induced in this loop current.
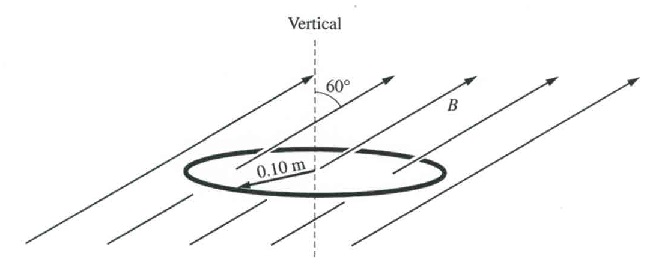
Question 5. A circular wire loop with radius 0.10 m and resistance 50 Ω is suspended horizontally in a magnetic field of magnitude B directed upward at an angle of 60o with the vertical. The magnitude of the field in teslas is given as a function of time t in seconds by the equation Show the direction of induced in this loop current and define the energy dissipated in the loop from t=0 to t=4 sec.
Question 6. The circuit consists of a battery of emf ε in series with a rod of length l, mass m, and resistance R. The rod is suspended by vertical connecting wires of length d, and the horizontal wires that connect to the battery are fixed. All these wires have negligible mass and resistance. The rod is a distance r above a conducting cable. The cable is very long and is located directly below and parallel to the rod. Earth’s gravitational pull is toward the bottom of the page. With the proper current in the cable Ic , the rod can be lifted up such that there is no tension in the connecting wires. Determine this current and magnetic flux through the circuit due to it.

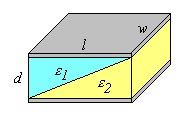
Question 7. A parallel plate capacitor with plates of area A and plate separation d has the region between the plates filled with two dielectric materials (ε1 and ε2) as shown in the figure. Determine the capacitance and show that when ε1= ε2= ε your result becomes the same as that for a capacitor containing a single dielectric:
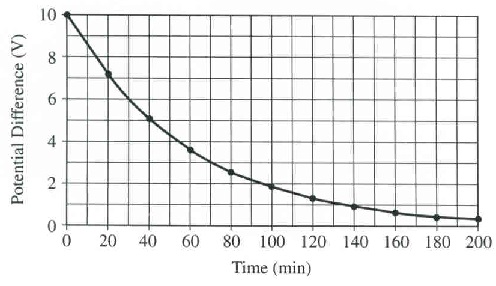
Question 8. You have been hired to determine the internal resistance of 8.0 μF capacitor for an electronic component manufacturer. (Ideal capacitors have an infinite internal resistance – that is, the material between their plates is a perfect insulator. In practice, however, the material has a very small, but nonzero, conductivity.) You cannot simply connect the capacitors to an ohmmeter, because their resistance is too large for an ohmmeter to measure. Therefore you charge the capacitor to a potential difference of 10 V with a battery, disconnect it from the battery and measure the potential difference across the capacitor every 20 minutes with an ideal voltmeter, obtaining the graph shown. Using this graph determine the internal resistance of the capacitor.
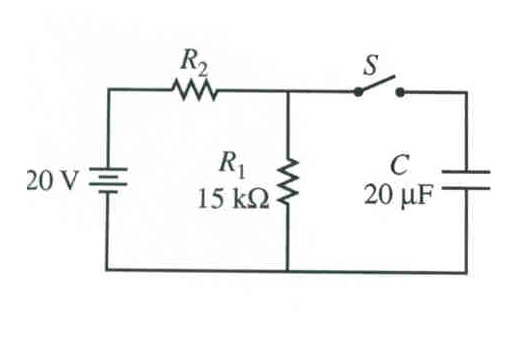
Question 9. In the circuit shown, the switch S is initially open and capacitor C is initially uncharged. The battery has an emf of 20 V negligible internal resistance. Resistor R1 has resistance of 15 kΩ and the capacitor C has a capacitance of 20 μF. Determine the voltage across resistor R2 immediately after and long time after the switch is closed. What energy is stored in the capacitor a long time after the switch is closed?
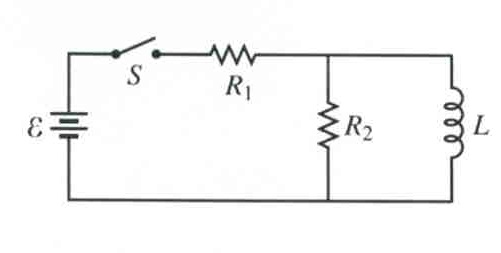
Question 10. In the circuit shown resistors 1 and 2 of resistance R1 and R2 respectively, and an inductor of inductance L are connected to a battery of emf ε and a switch S. The switch is closed at time t = 0. Sketch a graph of the current through the battery as a function of time.
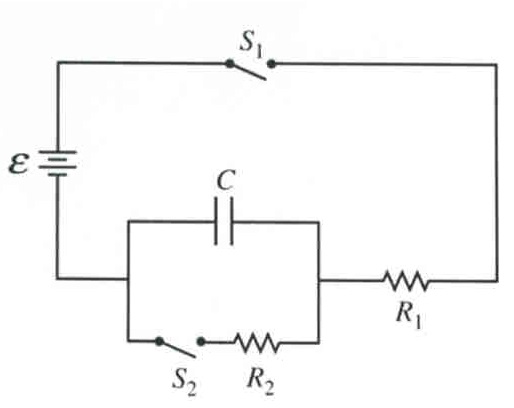
Question 11. The circuit contains a capacitor C, a power supply ε, two resistors of resistances R1 and R2, and two switches S1 and S2. Initially, the capacitor is uncharged and both switches are open. Switch S1 then gets closed at time t = 0.a) Write a differential equation that can be solved to obtain the charge on the capacitor as a function of time t. b) After switch S1 has been closed for a long time, switch S2 gets closed at new time t = 0. Define in this case currents I1 and I2 through resistors R1 and R2 respectively.
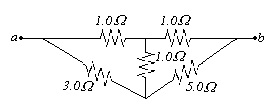
Question 12. For the network shown in the figure, prove that the resistance Rab = (27/17)Ω .
Question 13. An L-R-C series circuit is connected to an AC-source of constant voltage amplitude ΔU0 and variable angular frequency ω. a) At what angular frequency is the voltage amplitude across the resistor R at maximum? b) At what angular frequency is the voltage amplitude across the capacitor C at maximum? c) At what angular frequency is the voltage amplitude across the inductor L at maximum?
Question 14. Electromagnetic radiation is emitted by accelerating charges. The rate at which energy E is emitted from charge q moving with acceleration a is given by

,where c is the speed of light. a) Verify that this equation is dimensionally correct. b) If a proton with a kinetic energy of 6 MeV is traveling in a particle accelerator in a circular orbit of radius 0.75 m, what fraction of its energy does it radiate per second? c) If the electron in a hydrogen atom can be considered to be in a circular orbit with radius of 0.0529 nm and kinetic energy of 13.6 eV, how much energy would it radiate per second? (What does it tell you about the use of classical physics in describing the atom?)
Question 15. Electromagnetic waves propagate much differently in conductors than they do in dielectrics and vacuum. If the resistivity ρ of the conductor is sufficiently low (that is, if it is a sufficiently good conductor), the oscillating electric field of the wave gives rise to an oscillating conduction current that is much larger than the displacement current. In this case, the wave equation for an electric field E(x,t) = Ey(x,t) j propagating in the +x-direction within a conductor is given by
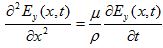
, where μ is the permeability of the conductor. a) Prove that solution for this equation is
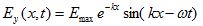
and define k. b) Explain why electric field exponentially decreases in amplitude as it propagates in the conductor. c) Show that the electric filed amplitude deceases by a factor 1/e in a distance 1/k and calculate this distance for a radio wave with frequency f = 1 MHz in copper (resistivity 1.72x10-8 Ω*m; permeability μ = μ0). Since this distance is so short, electromagnetic waves of such frequency can hardly propagate at all into copper. Instead, they are reflected at the surface of the metal. This is why radio waves cannot penetrate through copper or other metals, and why radio reception is poor inside a metal structure.