Problems:
Parametric equations and matrices.
The diagram below shows a line defined by the parametric equations , which crosses the x- and y-axes at the points (a, 0) and (0, b), respectively. The region marked A, is bounded by this line, the x- axes, the y- axes and the line x = u (with 0 £ u £ a ).
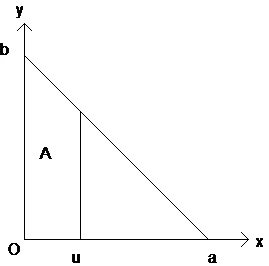
a) From the parametric equations , deduce the equation of the line in the form y = mx + c.
b) Find the values of a and b.
c) Determine an expression for the area A of the bounded region, in terms of u.
i) Find the completed square form of the expression in part (c).
ii) By using the expression in part (d) (i), or otherwise, write down the value of u which gives the maximum value of A.
iii) Write down this maximum value of A, and explain briefly why your answer makes sense from a geometrical point of view.
e) For what value of u is the value of A equal to half of the maximum area? Express your answer in surd form.
f) Consider the simultaneous equations:
12x + y = 18
-4x + 7y = 5
i) Write the equations above in the matrix form
Where A is a matrix and b is a 2 x 1 matrix.
ii) Find A-1, the inverse of matrix A.
iii) Use A-1 to solve the simultaneous equations.
iv) Explain the geometrical significance of the solution that you found in part (f) (iii).