Assignment:
Question 1. A harmonic force [F0 sin(ωt)] is applied to mass m1 which in turn is coupled to springs of spring constants k1, k2 and k3. Let x1 and x2 be the deviations from equilibrium for m1 and m2, respectively. Ignore gravity.
1) Write the equations of motion. Use x1 and x2 as your variables.
2) Find the normal frequencies of the system, that is, when the external force is absent. Assume for this part and the remainder of the problem the springs are the same and the masses are equal.
3) Solve for the steady state motion, that is find x1 and x2 as functions of time. (Hint: Assume complex solutions.)
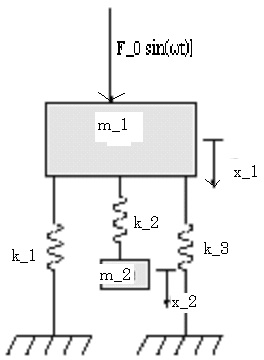
Just to clarify, in this graph, the masses and springs are all riding on a frictionless plane. Nothing is vertical and gravity is thus not involved.