Modi method and vogels approximation method

Question

Question 1:

a) What do you mean by the linear programming problem? Describe the steps comprised in linear programming problem formulation?

b) A paper mill generates two grades of paper viz., X and Y. Since of raw material restrictions, it can’t produce more than 400 tons of grades X paper and 300 tons of grade Y paper in a week. There are 160 production hrs in a week. It needs 0.20 and 0.40 hours to generate a ton of grade X and Y papers. The mill earns a gain of Rs. 200 and Rs. 500 per ton of grade X and Y paper correspondingly. Formulate this as a Linear Programming Problem.

Question 2:

a) Discuss the methodology of the Operations Research?

b) Illustrate in brief the phases of the Operations Research?

Question 3: Solve the following Linear Programming Problem by using Simple method.

Maximize Z= 3x₁ + 2X₂
Subject to the constraints:

                 X₁+ X₂ ≤ 4
                 X₁ - X₂ ≤ 2
                 X₁, X₂ ≥ 0

Question 4: Describe the procedure of MODI method of finding solution via optimality test.

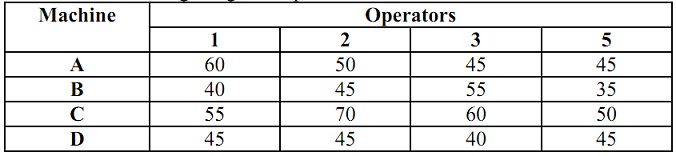
Question 5:

a) Describe the steps in the Hungarian method.

b) Solve the given assignment problem.

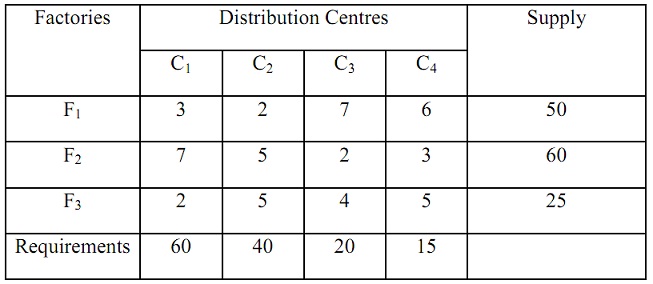
Question 6:

a) Describe the steps included in Vogel’s approximation method (VAM) of solving Transportation Problem.

b) Solve the given transportation problem by using the Vogel’s approximation method.