Assignment:
A. True/False Explain. Indicate whether each of the following statements is true or false and then explain why you think this. Include in your explanation any pertinent institutional details and economic reasoning (including appropriate graphs and equations). Please provide concise, clear answers with minimalir relevant detail. Explanation is required.
1. In the basic model of demand for insurance in competitive markets, if insurance is actuarially unfair consumers may prefer partial insurance to full insurance.
B. Analytical Problems
2. An individual has a health insurance plan with a deductible of $4800 and a coinsurance rate of 50%. Their demand curveis Q=30-(P/15), and the equilibrium market price of medical care is $300 per unit. What quantity of medical care would the individual choose to consume?
3. Consider a version of the Akerl of model in which neither buyers nor sellers observe car quality (though some how-please suspend your disbelief-both buyers and sellers enjoy higher utility from higher quality cars). For this question, please assume that both buyers and sellers recognize that neither can observe car quality.
Sellers' utility function is given by US = M + ∑ xi and buyers' utility is given by UB = M+∑3 xi where Mis the level of consumption of non-car goods and xi is the quality level of car, and there is a uniform distribution of quality of the cars held by sellers, xi~U[0,100].
In this market, is there is a price, p, at which all cars will sell? If not,prove there is no such price. If so,calculate what prices will work.
4. Demand for Insurance.The next two questions refer to Figure 2 below.
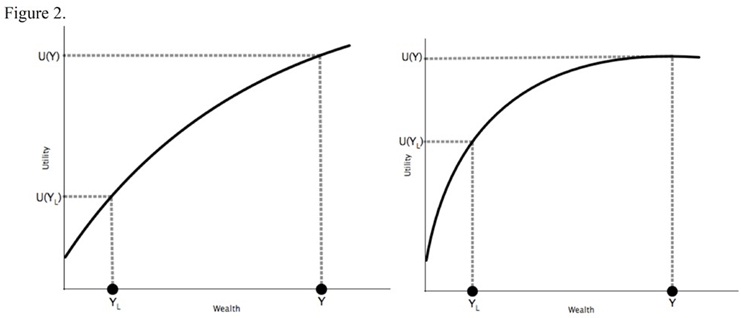
Suppose individual A and B both have a ½ probability of receiving Y and a ½ probability of receiving YL. Individual Ais more risk a verse than individual B.
a. Label which of the graphs above describes individual A and individual B' s utility function.
b. Suppose the insurer offers an actuarially unfair, full-insurance contract. Draw on each of the graphs the maximum administrative costs that each individual would be willing to pay. Is this amount larger for person A or person B?