Problem 1. Suppose that Jenny is taking two classes this semester: Biology and Economics. She knows that a class is only worth as much as you put into it so she is trying to get the maximum benefit from both classes. Her utility from taking these classes depends on the number of hours she spends each day studying, and is given by the following table:
a. Which class does Jenny prefer? (If she could only take one, which would it be?)
b. If Jenny has decided to spend 5 hours a day studying, what are the possible ways that she could split up her time? (Assuming she can only split her study time evenly into hours.)
c. What is her total utility from each of these options? Which option is optimal? Does this surprise you?
d. Now write down a table showing Jenny’s marginal utility of spending an additional hour studying each subject. What is true about the marginal utility of studying each class at the optimal allocation?
Problem 2. Timmy likes to eat apples and bananas. Suppose that each week Timmy spends all his weekly income on these two fruits.
a. If Timmy makes $20/week and apples cost $1 while bananas cost $0.50, what is Timmy’s budget constraint? Derive the equation and graph it with apples on the x-axis.
b. If he consumes both apples and bananas, what must his marginal rate of substitution be at the optimal consumption point? Assume that apples are on the x-axis.
c. Now suppose that there is serious inflation that causes the price of everything to double and Timmy’s income to double as well. Find Timmy’s new budget constraint. What will happen to his optimal consumption bundle? Describe what has happened in terms of real and nominal prices.
Problem 3. Define the following:
a. Income effect
b. Substitution effect
Problem 4. Suppose Ted consumes two goods, pieces of bread and slices of ham. Ted always makes sandwiches out of these two goods using two pieces of bread and three slices of ham. Ted only cares about how many of these sandwiches he can make.
a. Graph a few of Ted’s indifference curves.
b. If Ted has $28, bread costs $0.25 per piece and ham costs $0.50 per slice, what is the equation for Ted’s budget constraint if bread is the good measured on the y-axis? Graph it.
c. Given Ted’s budget constraint from part (b) what consumption bundle will Ted choose? How many sandwiches will this allow him to make?
d. Now suppose the price of ham increases to $1.00 per slice. Graph Ted’s new budget constraint and find his new optimal bundle.
e. Looking at Ted’s demand for bread, split his change in demand from part (c) to part (d) into the income and substitution effects.
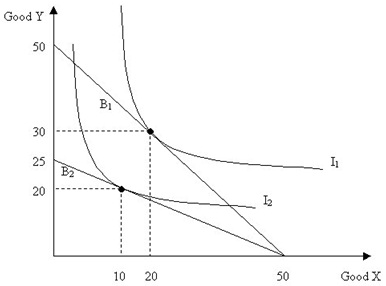
Problem 5. Below is a graph of a consumer’s budget constraint under two sets of prices, with income held constant at $150. Also drawn are the consumer’s indifference curves that are tangent to each of the budget constraints. Find this consumer’s demand curve for good Y. (Assume the demand is linear.)