Laplace transforms and transfer functions

Question

Assignment:

Compute the transfer function H(s) of the continuous-time system below given by the input/output differential equation.

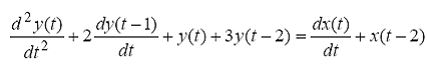
By using the Laplace transform, compute the convolution x(t)*v(t) of the two signals where x(t)=e^-tu(t) and v(t)=(sin t)u(t):

Using the convolution equation, I had x(t)*v(t) <-> X(s)V(s).

x(t)=e^-tu(t) <-> 1/s+1

for v(t)=(sin t)u(t),  I had s/s2 with ω = 1, an I came up with X(s)V(s) = s/( s³+s²) The choices are as follows:

• 1/2e^-t+1/2cos(t+45)     
• ½(e^-t-cost+sint)       
• (e^-t+cost-sint)      
• e^-t+1/2cost-1/2sint

Please assist in this calculation.

Determine the final value of: X(s) = (3s²+4s+1)/(s⁴+3s³+3s²+2s)

•  0
• 1/2
• infinity
• 8/9