Assignment:
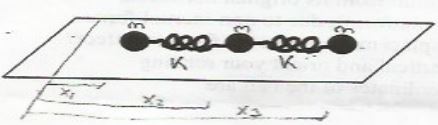
Three identical masses m are connected to 2 identical springs of stiffness k and equilibrium length b, as shown in the figure above. The masses are free to oscillate in one dimension along an axis that runs through all three. They lie on a level, frictionless, horizontal surface. Introduce coordinates, x1, x2 and x3 to measure their displacement from some fixed point on this axis.
Express the Lagrangian of the system in terms of these coordinates and their velocities. Then introduce the coordinates n1, n2, and n3, that measure the displacements of the springs from their equilibrium positions. Write the Lagrangian in terms of these coordinates and their time derivatives. Then find the kinetic and potential energy matrices. What are the frequencies of vibration for the normal modes of this system? What are eigenvectors?