Integrals in the sense of the lebesgue

Question

All the integrals below are understood in the sense of the Lebesgue.

Question 1: Prove the following equality which we used in class without proof. Assume that f integrable over [ 3; 3]. Then

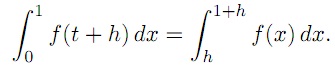
for any h ε (-3, 2).

Question 2: Assume that f is integrable over [0, 1]. Show that

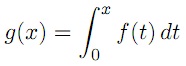
is dierentiable a.e on (0, 1).

Question 3: Assume that f is continuous on [0, 1]. Suppose that

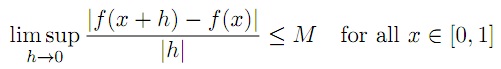
Then, |f(x) - f(y)| ≤ M |x-y|

for any x, y ε [0, 1]