Question 1 : Public program evaluation using Income and Substitution effect
Recalling the question, let us know interpret good X as units of bundles of food and good Y as units of bundles of luxury goods. Continue to assume that the consumer has their preferences for these two goods defined by the utility function u(X,Y) = XY. And, just like last week we can assume that the marginal utility of consuming X is given by Mux = Y and the marginal utility of consuming Y is given by Muy = X.
Use this information to answer the following questions.
a) Suppose initially that price of a unit of a food bundle is $5 and the price of a bundle of luxurious goods is $10. Determine the optimal combinations of food bundles and luxurious goods bundles for this individual if her income available to spend on these two types of goods is equal to $100. Graphically illustrate the optimal bundle (let’s call this combination of X and Y bundle A) in a well labeled graph; makes sure you indicate where the optimal consumption bundle A is located. Show the work you did to find your answer.
b) Suppose that the individual’s income and the prices of both types of goods increase proportionately: for example, assume that income and the prices of both types of goods all triple. Compared to your answer in (a), how does this change in income and prices affect the optimal consumption bundle?
c) Suppose that income increases by three times the initial amount while the prices of good X and good Y stay at their initial level. Given these changes, what will happen to the new optimal consumption bundle? Given your result , can one of these two goods be a Giffen goods (hint: you might find it helpful to read in your text about Giffen goods - page 284)? Justify your reason. (Note: th is question should be answered based solely on the result that you find in this question. No need to answer the next question and then return to this question. )
d) Return to the initial situation described in (a). Now, suppose that government imposes a $5 per unit tax on each food bundle purchased. Recalculate the optimal combination bundle for these two goods given this new tax. Then, determine the tax revenue that the government will raise from this individual .
e) Draw a new graph of this individual’s budget line and optimal consumption bundle from (a) as well as the new budget line and optimal consumption bundle once the tax per unit on good X is implemented by the government (you found this in (d)). Label the new budget line BL2 and the new optimal consumption bundle with the tax point B. Explain in your own word s and with a 2 step - by - step procedure how you can isolate the substitution effect from the income effect. For this problem, it might also be a good idea to c alculate the bundle “ C ” on the imaginary budget line.
f) Given the new price of good X which includes the tax per unit on good X, how much money would the government need to give this individual to bring her back to her original level of satisfaction that she had before the tax on good X was implemented? If the amount of income compensation the government must make to the individual exceeds the total revenue the government collects from this individual with the tax on good X , what does this imply to you in term s of the welfare loss/gain of tax policy?
Question 2: Production and cost analysis
Suppose that q is the number of patients that a hospital can serve per day. The number of patients served per day depends on two things: first, the number of doctors hired to practice in each day (L) , and second the number of diagnostic machines (K) installed in the medical laborator y. The table below summarizes the production and cost functions for the hospital.

a) Fill in the number for each cell in the table that has been left blanked. Make sure that the number s that you fill in are consistent with one another. Given all the numbers that you’ve filled in, answer the following questions.
b) Is the hospital now operating under short - run or long - run production technology? Explain your answer.
c) What is the price of a unit of capital and the price of a doctor?
d) Find the range of output that minimize s average total cost. e) Does the law of diminishing marginal productivity of labor hold given the technology of this hospital? Explain your answer.
f) Suppose that the market price of medical service that each hospital can charge to each patient is $ 65. How many doctor s will you hire if you wish to maximize the economic profit of the hospital? How much profit does the hospital e arn when it profit maximizes? Explain your answer.
g) Suppose that the price the hospital can charge each patient falls to $21 per patient. Should the hospital continue to operate in the industry in the short - run given this price decrease? Explain your answer.
Question 3 : Perfect competition and monopoly
Answer the following questions using the table below.
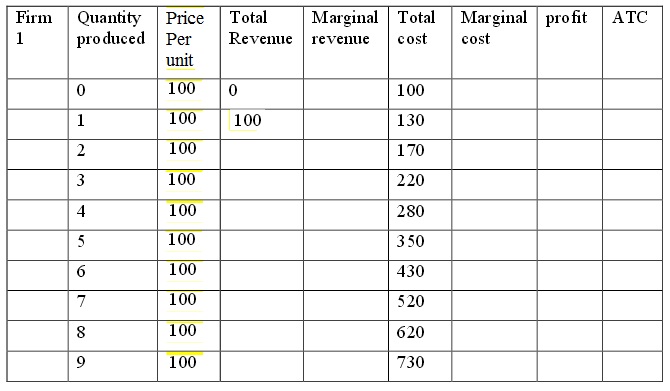
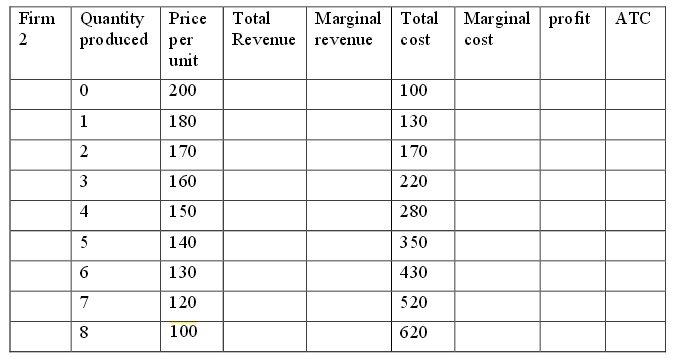
a) Complete the table for the two firms above.
b) What is the profit - maximizing output for each firm? (Hint: remember that profit maximization requires MR = MC)
c) What is the relationship between price, marginal revenue and marginal cost at the profit maximizing level of output for each firm?
d) Which firm is in a competitive market, which is a monopolist an d how do you know? Explain your answer .
e) What is the dollar amount of the dead weight loss for the monopolist? Explain your answer.
Question 4 : Monopoly
Suppose there is a monopoly that mines diamonds. The marginal revenue and marginal cost functions for this monopolist are given by the following equations:
MC = 2 + 2q
MR = 10 - 2q
(a) Given the above information, write the monopolist’s demand curve for diamonds in y - intercept form ? Assume this demand curve is linear.
(b) Given the above information, w hat is the profit - maximizing level of output for this monopolist?
(c) What price should the monopolist charge when it produces the profit maximizing quantity? Explain your answer.
(d) Suppose you know that the monopolist’s total costs can be calculated using the equation TC = 2 + 2q + q*q . Given this information, write equations for TFC, TVC, and ATC.
(e) Given the above information and the work you have done, now calculate the monopolist’s economic profit. How much profit/loss does the firm earn? Show how you calculated this answer.
(f) Suppose the monopolist’s fixed costs change and they are now equal to $8 . How would this change the firm’s TC equation? What is the economic profit for the monopolist given this change ? Would your answer change if the fixed costs were to change to $10? Explain your answer fully.
(g) What does this tells us about a monopolist's profit and the costs of obtaining a monopoly?
Question 5 : Monopoly
Use the following graph for a monopoly to answer this question.
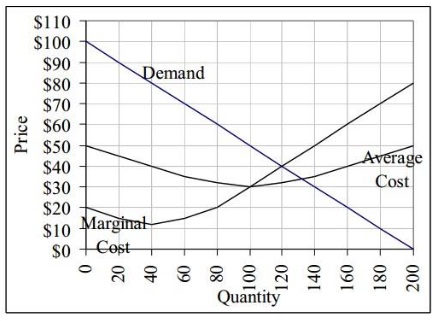
a) What will be the price and quantity sold for the monopoly? How much is the monopoly earning in profits? Explain your answer fully using a step - by - step approach.
b) Based on your answer to part (a) , explain in words what you think will happen in the long run.
c) Is the market efficient with the monopoly? If so, explain how you know this. If not, state what quan tity would be economically efficient and why that quantity would be economically efficient.
Question 6 : Perfect competition in short run and long run Suppose a firm sells its product in a perfect ly competitive market where all the firms in the market charge a price of $40 per unit for the good . The firm’s total cost is TC = 40 + 8q – 2 q2 + q3 and the firm’s MC = 8 – 4q + 3 q 2 .
a) How much output should the firm produce in the short run? Explain your answer.
b) What price should the firm charge in the short run? Explain your answer.
c) What are the firm’s short - run profits? Explain your answer.
d) Given your answers in (a) through (c), what do you predict will happen in this market in the long run? Explain your answer.
e) Draw a graph that represents this firm’s average variable cost , average total cost and marginal cost for q be tween 0 and 5. Draw all curves in the same figure and as exactly as possible. How can you determ ine the long run market price for this perfectly competitive firm’s product graphically?
Question 7: Perfect competition Assume that the taxi industry in the town of New City is perfectly competitive. Also assume that the marginal cost of a taxi ride is constant and equal to $5 per trip (this assumption will make the math far simpler for thi s question) and that each taxi is capable of making 20 trips a day. We will let the demand function for taxi rides each day be Q = 1,100 – 20P.
a) What is the perfectly competitive price of a taxi ride?
b) How many rides will the citizens of New City make every day?
c) How many taxis will operate in New City?