Complete the following:
Q1. The driving distance for the top 100 golfers on the PGA tour is between 284.7 and 310.6 yards (Golfweek,March 29,2003). Assume that the driving distance for these golfers is uniformly distributed over this interval.
a. Give a mathematical expression for the probability density function of driving distance.
b. What is the probability the driving distance for one of these golfers is less than 290 yards?
c. What is the probability the driving distance for one of these golfers is at least 300 yards?
d. What is the probability the driving distance for one of these golfers is between 290 and 305 yards?
e. How many of these golfers drive the ball at least 290 yards?
Q2. Suppose we are interested in bidding on a piece of land and we know one other bidder is interested. The seller announced that the highest bid in excess of $10,000 will be accepted. Assume that the competitor's bid x is random variable that is uniformly distributed between $10,000 and $15,000.
a. Suppose you bid $12,000. What is the probability that you bid will accepted?
b. Suppose you bid $14,000. What is the probability that you bid will accepted?
c.What amount should you bid to maximize the probability that you get the property?
d. Suppose you know someone who is willing to pay you $16,000 for the property. Would you consider bidding less than the amount in part (c)? Why or why not?
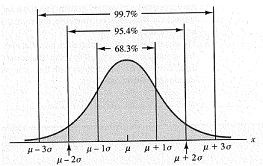
Q3. A random variable is normally distributed with a mean of µ=50 and a standard deviation of
a) a. Sketch a normal curve for the probability density function. Label the horizontal axis with values of 35,40,45,50,55,60 and 65. The figure below shows that the normal curve almost touches the horizontal axis at three standard deviations below and at three standard deviations above the mean (in this case at 35 and 65).
b) What is the probability the random variable will assume a value between 45 and 55?
c) What is the probability the random variable will assume a value between 40 and 60?
Q4. Given that z is a standard normal random variable, compute the following probabilities.
a) P(-1 <= z <= 0)
b) P( -1.5 <= z <= 0)
c) P( -2 <= z <= 0)
d) P(-2.5 <= z <= 0)
e) P( -3 <= z <= 0)
Q5. The time needed to complete a final examination in a particular college course is normally distributed with a mean of 80 minutes and a standard deviation of 10 minutes. Answer the following questions.
a) What is the probability of completing the exam in one hour or less?
b) What is the probability that a student will complete the exam in more than 60 minutes but less than 75 minutes?
c) Assume that the class has 60 students and that the examination period is 90 minutes in length. How many students do you expect will be unable to complete the exam in the allotted time?