Assignment:
Q1. Show that, if
(a) a fair die is thrown times independently, it is more likely than not that at least one six appears;
(b) a pair of fair dice are thrown 24 times independently, it is more likely than not that a double six does not appear.
(This pair of calculation has an honoured place in the history of the development of the formal study of probability. Some seventeenth century gamblers are said to have believed that, sin (a) holds, then having six times as many throws (4-→ 24) “ought” to give the same chance of getting an event that was one sixth as likely (six -→ double six). It is very satifying to see a loose argument give the wrong answer).
Q2. In the diagram, the numbers are the (independent) chances the components will fail within ten years. Find the chance the system fails with ten years.
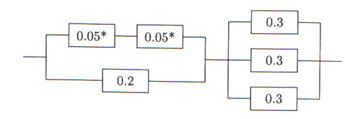
Given that the system has not failed in ten years, find the chance neither component marked * has failed.
Q3. Poker die have six equally likely faces labeled { 9,10,J,Q,K,A}. When five such dice are thrown independently, what are the probabilities of the different types of “hand” which, in rank order are Five of a Kind(aaaaa); Four of a Kind(aaaab); Full House(aaabb); Three(aaabc); Two Pairs(aabbc); One Pair(aabcd); and no Pair(abcde)?
(“Run” are not normally considered in this game.)
Given any of these hands, you are allowed to roll again any dice that unmatched. Find your repective chances of improving your hand for the different initial holding (ignoring “improvements” such as turning (KKKQJ) to (KKKA9).
Provide complete and step by step solution for the question and show calculations and use formulas.