Suppose that your company has finished designing a new product. The company now wants to establish a distribution network for the markets it can sell the product. The product will be sold for $15 per unit in any market. There are 3 potential markets that the company can choose to enter. If the company chooses to enter the market, the demand in that market should be satisfied. That is, the number of products shipped to the market should be exactly equal to the demand of the market. Therefore, the potential revenues that can be gained by entering a market is equal to the demand of the market multiplied by the per unit selling price of the product. The table below specifies the demand for each market.
To manufacture the product, so that the demand in the chosen markets can be satisfied, the company needs to decide on the locations of the manufacturing facilities. There are two possible locations for locating manufacturing facilities. The table below gives the cost of locating a manufacturing facility and the capacity of the located manufacturing facility for each possible location.
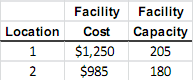
The company needs to ship the product to the chosen markets from the located manufacturing facilities. Any market can be supplied by any manufacturing facility. For instance, if the company chooses to enter market 1 and locates manufacturing facilities in locations 1 and 2, the demand for market 1 can be shipped partially from manufacturing facility in location 1 and manufacturing facility location 2. The per unit shipping cost from each facility location to each market is given in the table below.

As the planning manager of the company, you are required to determine which markets to enter, where to locate manufacturing plants, and how much to ship from each located manufacturing plant to each chosen market to maximize the total profit the company makes. The total profit is equal to the revenues gained from selling the product in the chosen markets minus the manufacturing facility location and shipping costs. (The quantities shipped can be continuous, i.e., they do not have to be integer.)
Mathematically formulate a mixed integer linear programming model for the problem stated above. (i) Define your decision variables, (ii) formulate the objective and the objective function in terms of your decision variables, (iii) formulate the constraints and explain why they are needed, and (iv) combine everything to have the final mathematical formulation.