Assignment:
(a) The odd function f(x) of period L may be expanded as

Give expressions for kn and An. Given that f(x) = 1 for 0 < x < L/2, obtain an expression for An. Hence, or otherwise, show that
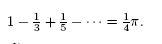
(b) The Fourier transform f(k) of a non-periodic function f(x) is defined to be
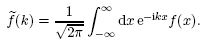
write down the inverse transform. The function f(t, x) satisfies the partial differential equation
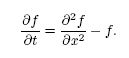
Show that the Fourier transform
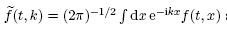
satisfies

Given the initial condition
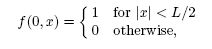
show that

Provide complete and step by step solution for the question and show calculations and use formulas.