Assignment:
Signaling games This exercise illustrates that a college education serves as a form of signaling to potential employers, in addition to expanding the knowledge of students. A young person entering the job market may be talented or untalented. Suppose that one-quarter of high school graduates are talented, and the rest untalented. A recent high school graduate, who knows whether or not he is talented, has the option of spending a year traveling overseas or enrolling at college (we will assume that he or she cannot do both) before applying for a job. An employer seeking to fill a job opening cannot know whether or not a job applicant is talented; all he knows is that the applicant either went to college or traveled overseas. The payoff an employer gets from hiring a worker depends solely on the talents of the hired worker (and not on his educational level), while the payoff to the youth depends on what he chose to do after high school, on his talents (because talented students enjoy their studies at college more than untalented students), and on whether or not he gets a job. These payoffs are described in the following tables (where the employer is the row player and the youth is the column player, so that a payoff vector of (x, y) represents a payoff of x to the employer and y to the youth)
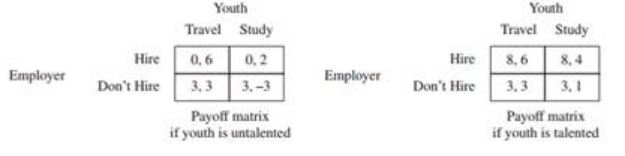
(a) Depict this situation as a Harsanyi game with incomplete information.
(b) List the pure strategies of the two players.
(c) Find two Bayesian equilibria in pure strategies.
Provide complete and step by step solution for the question and show calculations and use formulas.