Solved: Find the joint distribution of the random sample in part c, Engineering Mathematics

+44 141 628 6080
[email protected]

Find the joint distribution of the random sample in part c

1. For each of the following distributions, find the expected value of X.

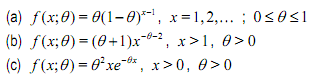
690_Find the joint distribution of the random sample.png

2. Let X₁,......X_n be a random sample from each of the distributions in problem 1. In each case, find the joint distribution of X₁,......X_n.

3. The amount of time (in hours), , X needed by a local repair shop, Rusts 'R Us, to repair a randomly selected piece of equipment is assumed to be an exponential random variable with pdf

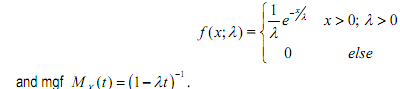
2094_Find the joint distribution of the random sample1.png

(a) Explain in words what the random variable, , X represents in this scenario. Why is X considered a random variable?

(b) Identify the parameter and explain in words what the parameter represents in this scenario.

14, 6, 3, 4, 1.5

Explain in words what n, X₁,......X_n and x₁,......x_nare in this situation, and, if applicable, give the numerical values of each.

(d) Find the joint distribution of the random sample in part (c). List all assumptions made when finding this joint distribution and explain whether or not each is reasonable in this scenario.

(e) Rusts 'R Us wants to estimate the expected amount of time needed to repair a piece of equipment. Identify the parameter Rusts 'R Us wants to estimate, and explain why the sample mean, , X is an appropriate statistic for the business to use.

(f) Why is the statistic identified in part (e) considered a random variable? Give the observed value of the statistic, , x based on data provided in part (c).

Attachment:- Assignment.rar

View Complete Question

Solution Preview :

Prepared by a verified Expert

Engineering Mathematics: Find the joint distribution of the random sample in part c

Reference No:- TGS0619418

Now Priced at $40 (50% Discount)

Recommended (96%)

Rated (4.8/5)

Have a Question? (oR Write a Review)

Write atleast 100 words!!

Recent Questions Asked Engineering Mathematics

Q : Purified a new protein and find that it contains 0154

purified a new protein and find that it contains 0.154 percent Mn. Assuming that there are three atoms of manganese per molecule of protein, what is the molar mass of the protein

Q : A rom chip of 1024 x8 bits has four select inputs and

A ROM chip of 1024 X8 bits has four select inputs and operates fron a 5 volt power supply. How many pins are needed for the IC package. Draw a block diagram and label all input and output terminals in the ROM.

Q : Explain why light intensity is dependent on the distance

Explain why light intensity is dependent on the distance the detector is from the light source. Describe the mathematical relationship. Explain why all comparative measurments for this experiment should be made with the optical cable the same dist

Q : If 200 g of water is contained in a 300g aluminum

If 200 g of water is contained in a 300g aluminum calorimeter at 20°C and 20g of steam at 100°C is introduced into the calorimeter

Q : Find the joint distribution of the random sample in part c

Find the joint distribution of the random sample in part (c). List all assumptions made when finding this joint distribution and explain whether or not each is reasonable in this scenario.

Q : Create a procedure of not more than 5 steps that you think

Create a procedure of not more than 5 steps that you think will work to compare the efficiency of the light emitted by an incandescent light bulb to that emitted by a fluorescent light bulb. Make sure to mention all the variables you think you'll

Q : The binary-coded-decimal bcd format uses 4 bits to

The binary-coded-decimal (BCD) format uses 4 bits to represent 10 decimal digits. For example, 259 in base 10 is repesented as "010 0101 101" in BCD format. A BCD incrementor adds 1 to a number in BCD format.

Q : A planet of mass m 815 atilde 1024 kg is orbiting in a

A planet of mass m = 8.15 Ã? 1024 kg is orbiting in a circular path a star of mass M = 6.05 Ã? 1029 kg. What is the orbital period (in earth days) of the planet Tplanet

Q : An isolated free electron is traveling through an electric

An isolated free electron is traveling through an electric field from some initial point where its coulombic potential energy per unit charge (voltage) is 17 kJ/C and velocity = 93 Mm/s to some final point where its coulombic potential per unit ch

1955321

Questions
Asked

3,689

Active Tutors

1423610

Questions
Answered

Start Excelling in your courses, Ask a tutor for help and get answers for your problems !!

ask Question

Solution Preview :

Prepared by a verified Expert

Engineering Mathematics: Find the joint distribution of the random sample in part c

Reference No:- TGS0619418

Have a Question? (oR Write a Review)

Recent Questions Asked Engineering Mathematics

Q : Purified a new protein and find that it contains 0154

Q : A rom chip of 1024 x8 bits has four select inputs and

Q : Explain why light intensity is dependent on the distance

Q : If 200 g of water is contained in a 300g aluminum

Q : Find the joint distribution of the random sample in part c

Q : Create a procedure of not more than 5 steps that you think

Q : The binary-coded-decimal bcd format uses 4 bits to

Q : A planet of mass m 815 atilde 1024 kg is orbiting in a

Q : An isolated free electron is traveling through an electric

What when people get involved in community and social change

Problem regarding culturally relativistic

How code of suburbs differs from the code of street

Why does victor coin the term hyper-violence

Examine gender inequality on a global scale

Which of the things happened first

Which is a criticism of marxist/conflict theories

Solution Preview :

Prepared by a verified Expert

Engineering Mathematics: Find the joint distribution of the random sample in part c

Reference No:- TGS0619418

Recent Questions Asked Engineering Mathematics

Q : Purified a new protein and find that it contains 0154

Q : A rom chip of 1024 x8 bits has four select inputs and

Q : Explain why light intensity is dependent on the distance

Q : If 200 g of water is contained in a 300g aluminum

Q : Find the joint distribution of the random sample in part c

Q : Create a procedure of not more than 5 steps that you think

Q : The binary-coded-decimal bcd format uses 4 bits to

Q : A planet of mass m 815 atilde 1024 kg is orbiting in a

Q : An isolated free electron is traveling through an electric

Asked Questions