Solve the following problem:
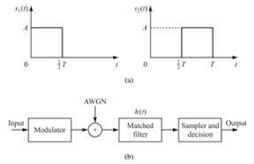
A baseband digital communication system employs the signals shown in Figure (a) for the transmission of two equiprobable messages. It is assumed that the communication problem studied here is a "one-shot" communication problem; that is, the above messages are transmitted just once and no transmission takes place afterward. The channel has no attenuation (α = 1), and the noise is AWGN with power spectral density ½N0.
a. Find an appropriate orthonormal basis for the representation of the signals.
b. In a block diagram, give the precise specifications of the optimum receiver using matched filters. Label the diagram carefully.
c. Find the error probability of the optimum receiver.
d. Show that the optimum receiver can be implemented by using just one filter (see the block diagram in Figure (b)). What are the characteristics of the matched filter, the sampler and decision device?
e. Now assume that the channel is not ideal but has an impulse response of c(t) = δ(t) + 1 2 δ(t - ½T ). Using the same matched filter as in (d), design the optimum receiver.
f. Assuming that the channel impulse response is c(t) = δ(t) + aδ(t - ½T ), where a is a random variable uniformly distributed on [0, 1], and using the same matched filter as in (d), design the optimum receiver.