Assignment:
Q1. Functions f, g, and h are continuous and differentiable for all real numbers, and some of their values and values of their derivatives are given by the below table.
x f (x) g(x) h(x) f ′(x) g′(x) h′(x)
0 1 -1 -1 4 1 -3
1 0 3 0 2 3 6
2 3 2 4 - 2 1 3
a) Let F(x) = g(x) e^2 f ( x) , find F ′(0) .
b) LetG(x) = ln[g(x) / h(x)] , findG′ (2) .
c) Let H(x) = e[h( x)+ g ( x)]^2 , find H′(1) .
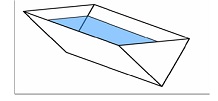
Q2. The 4-foot-long manger shown in the accompanying figure has a cross section which is an equilateral triangle with side length 18 inches. If rain is accumulating in the manger at the rate of 250 cubic inches/hour, how fast is the water level rising in the manger when it is 10 inches deep?
Q3. A satellite is in an elliptical orbit around the Earth. Its distance r (in miles) from the center of the Earth is given bt r= 4995/ 1 + 0.12cosθ, where θ is the angle shown in thefigure.
a) Find the altitude of the satellite at perigee (the point nearest to the surface ofthe Earth) and at apogee (the point farthest from the surface of the Earth) given the radius of the Earth is 3960 mi.
b) At the instant when θ = 120° , the angle θ is increasing at the rate of 2.7° /min. Find the altitude of the satellite and the rate at which the altitude is changing at this instant.
