Assignment:
Major Motors produces its Trans National model in three plants located in Flint, Michigan; Fresno, California; and Monterrey, Mexico. Dealers receive cars from regional distribution centers located in Phoenix, Arizona; Davenport, Iowa; and Columbia, South Carolina. Anticipated production at the plants over the next month (in 100s of cars) is 43 at Flint, 26 at Fresno, and 31 at Monterrey. Based on firm orders and other requests from dealers, Major Motors has decided that it needs to have the following numbers of cars at the regional distribution centers at month's end: Phoenix, 26; Davenport, 28; and Columbia, 30. Suppose that the cost of shipping 100 cars from each plant to each distribution center is given in the following matrix (in $ 1,000s):
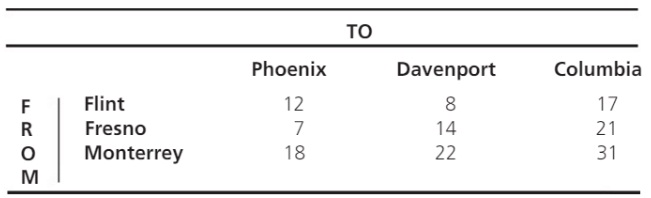
a. Convert the problem to a balanced problem by adding an appropriate row or column and find the optimal solution using Solver.
b. Now find the optimal solution using Solver and inequality constraints.
c. Do the solutions in (a) and (b) match? Why or why not?
d. Suppose that the route between Monterrey and Columbia is no longer available due to a landslide on a key road in Mexico. Modify the model in (b) and resolve to find the optimal solution. Has the objective value increased or decreased? Explain why.