Exponential and logarithm problems

Question

Assignment:

Q1. In the formula A = I^ekt , A is the amount of radioactive material remaining from an initial amount I at a given time t and k is a negative constant determined by the nature of the material. An artifact is discovered at a certain site. If it has 53% of the carbon-14 it originally contained, what is the approximate age of the artifact? (carbon-14 decays at the rate of 0.0125% annually.) (Round to the nearest year.)

• 4240 yr
• 5079 yr
• 2206 yr
• 3760 yr

Q2. Suppose the amount of a radioactive element remaining in a sample of 100 milligrams after x years can be described by A(x) = 100e^-0.01657x . How much is remaining after 257 years? Round the answer to the nearest hundredth of a milligram.

• 425.85 milligrams
• 7070.31 milligrams
• 1.41 milligrams
• 0.01 milligrams

Q3-Use a graphing calculator to predict about how many books will have been read in the eighth grade.

• 1000
• 2000
• 3000
• 500

Q4-Write the logarithmic and exponential equations associated with the display.

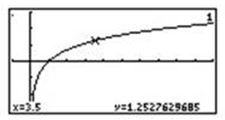
g(x) = ln x

• ln 3.5 = .54406804435; e^.54406804435 = 3.5
• ln .54406804435 = 3.5; e^3.5 = .54406804435
• ln 3.5 = 1.2527629685; e^1.2527629685 = 3.5
• ln 1.2527629685 = 3.5; e^3.5 = 1.2527629685

Q5-Write the logarithmic and exponential equations associated with the display.

f(x) = log x

• log .301029995664 = 2; 10² = .301029995664
• log .69314718056 = 2; 10² = .69314718056
• log 2 = .301029995664; 10^{.301029995664} =2
• log 2 = .69314718056; 10^.69314718056 = 2

Q6-Write the logarithmic and exponential equations associated with the display.
f(x) = log x

• log 4 = .602059991328; 10^{.602059991328} = 4
• log .602059991328 = 4; 10⁴ = .602059991328
• log 1.38629436112 = 4; 10⁴ = 1.38629436112
• log 4 = 1.38629436112; 10^{1.38629436112} = 4

Provide complete and step by step solution for the question and show calculations and use formulas.