Activity: Decision Making Models
Complete the following Activity:
Activity 1
If you were given a choice, which of the following gambles would you prefer?
1. $1,000,000 for certain
2. A 10 percent chance of getting $2,500,000, an 89 percent chance of getting $1,000,000, and a 1
Activity 2:
Suppose you consider the possibility of insuring some property against damage (e.g. fire or theft). After examining the risks and the premium you find that you have no clear preference between the options of purchasing insurance or leaving the property uninsured.
It is then called to your attention that the insurance company offers a new program called probabilistic insurance. In this program you pay half of the regular premium. In case of damage, there is a 50 percent chance that you pay the other half of the premium and the insurance company covers all of the losses; and there is a 50 percent chance that you get back your insurance payment and suffer all of the losses.
For example, if an accident occurs on an odd day of the month, you pay the other half of the regular premium and your losses are covered; but if the accident occurs on an even day of the month, your insurance payment is refunded and your losses are not covered.
Recall that the premium for full coverage is such that you find this insurance barely worth its cost. Under these circumstances, would you purchase probabilistic insurance?
Activity 3:
How much money would you pay to play a game in which an unbiased coin is tossed until it lands on Tails, and at the end of the game you are paid ($2.00)k where k equals the number of tosses until Tails appears? In other words, you would be paid $2.00 if Tails comes up on the first toss, $4.00 if Tails comes up on the second toss, $8.00 if Tails comes up on the third toss, and in general:
Tosses Until Tails 1 2 3 4 5 ... K
Payoff in Dollars 2 4 8 16 32 ... 2K
Activity 4
If you were given a choice which of the following would you prefer?
1. A 1 in 1000 chance of winning $5000.00
2. A sure gain of $5.00.
Activity 5:
You are the chair of a faculty search committee consisting of five other members: Ann; Bob; Cindy; Dan; and Ellen. Your task is to hire a new professor and the top three preferences have already been determined to be Joe Schmoe, Jane Doe, and Al Einstein. Suppose you know everyone else's preferences, as shown in the table, and you want to control the balloting so that Al Einstein is chosen.
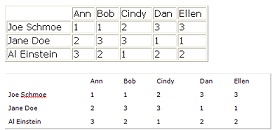
A.What should you do?
1. Call for a direct vote between Schmoe and Einstein followed by a direct vote between Einstein and Doe
2. Call for a direct vote between Schmoe and Doe followed by a direct vote between Schmoe and Einstein
3. Call for a direct vote between Einstein and Schmoe followed by a direct vote between Doe and Einstein
B. Why can the person setting the agenda have complete control over the outcome?