Explain distance between centres of circles

Question

Assignment:

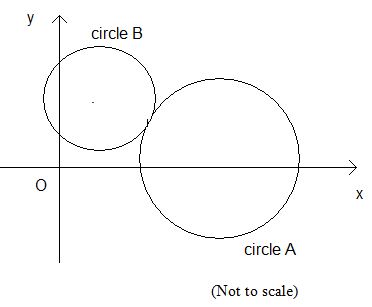
Two circles, A and B, touch each other at exactly one point, as shown in the diagram below. The equation of circle A is x² - 18x + y² - 2y + 46 = 0. Circle B has centre (1, k + 1) and radius 4.

a)

i) Find the completed square form of x² - 18x

Find the completed square form of y² - 2y

ii) Use your answer from part i) to express the equation of circle A in the form

(x - a)² + (y - b)² = r²

iii) Write down the centre and radius of circle A.

b)

i) Explain why the distance between the centres of circles A and B is 10.

ii) Express this distance also in terms of k, and hence find the two possible values of k.

iii) Hence show that the two possible equations of circle B are

(x - 1)² + ( y - 7)²  = 16 and (x - 1)² + ( y + 5)² = 16

c) Show algebraically that the line y = 4/3 x - 1 is a tangent to circle A, and give the coordinates of the point of contact.

Provide complete and step by step solution for the question and show calculations and use formulas.