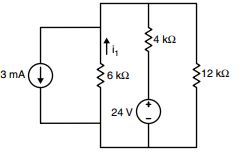
Discuss the below:
Q1: (a) Use the method of superposition to determine the current i1 in the above circuit.
(b) Now solve for i1 using the node-voltage method, and show that you get the same result.
(c) Based on your answer to (a) and (b), calculate how much power is dissipated in each of the three resistors in the circuit.
(d) Calculate how much power the voltage source is supplying/consuming.
(e) Calculate how much power the current source is supplying/consuming.
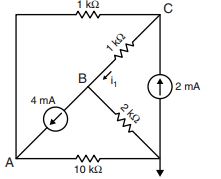
Q2: In the following circuit, the nodes have been labeled for you and a reference node has been selected.
(a) Use the node-voltage method to derive a set of linear equations that could be used to solve for the unknown node voltages vA, vB and vC in the above figurebCollect your equations into a matrix equation of the form: Ax = b where
x=[va vb vc]
(b) Solve the equations to obtain the node voltages. (You may use Matlab, your calculator, or other tools, but please check your result to make sure that it is self-consistent.)
(c) Determine the current i1.
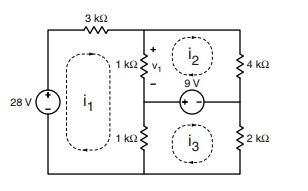
Q3: In the following circuit, the mesh-currents have been labeled for you.
(a) Use the mesh-current method to derive a set of linear equations that could be used to solve for the unknown mesh-currents i1, i2 and i3 in the above figure.
Collect your equations into a matrix equation of the form: Ax = b where
x ≡ [i1 i2 i3]
(b) Solve the equations to obtain the currents. (You may use Matlab, your calculator, or other tools, but please check your result to make sure that it is self-consistent.)
(c) Determine the voltage v1.
Q4: The following circuit can be solved using either the node-voltage or mesh-current method.
(a) Use the node-voltage method to derive a set of linear equations that can be used to solve for the two unknown node voltages vA and vB in the above figure. Collect your equations into a matrix equation of the form: Ax = b where
x ≡[vA vB]
(b) Now use the mesh-current analysis to derive a set of linear equations that can be used to solve for the mesh currents i1 and i2 in the same circuit. Collect your equations into a matrix equation of the form: Ax = b where
x ≡[ i1 i2]
(c) Solve for all of the unknown currents and voltages using either technique. You may use a calculator to check your answers, but you must solve the equations by hand and show work. Draw a large copy of the circuit and clearly indicate the values for all of the branch voltages and currents.
