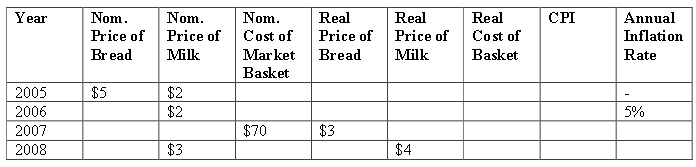Part I – Excise Taxes
Question 1. (You are not required to plot anything for this part, but, as usual, plotting provides valuable insight into both the calculations and the intuition.)
Suppose the market for hot chocolate in January in Madison can be described by the following supply and demand curves:
Supply: Qs = 5P – 5 for P ≥ 1
Demand: Qd = 100 – 10P for 0 ≤ P ≤ 10
where Qs is the quantity supplied, Qd is the quantity demanded, and P is the price.
a) Find the equilibrium quantity and supply in this market.
b) What is consumer and producer surplus in this market?
c) Using the point elasticity formula, calculate the price elasticities of demand and supply at the equilibrium point.
d) Suppose February is more bitterly cold than usual, increasing demand for hot chocolate by 60 units at any given pr ice. Supply remains as before. Given this information, w hat is the new equi librium price and quantity?
e) What is the new consumer and producer surplus, CS' and PS'?
f) What are the new price elasticities of supply and demand using the point elasticity formula?
g) Suppose the city of Madison decides to tax hot chocolate with a $3 per unit excise tax on suppliers. Calculate the equilibrium quantity (Qet), price (Pet), consumer surplus (CSt), producer surplus (PSt), government revenue (Govt. Tax Rev.) , tax incidence on consumers (CTI), tax incidence on producers (PTI) , and deadweight loss (DWL) from this tax for January (before the change in demand)
h) What is the ratio of the absolute value of the slope of the demand curve to the slope of the supply curve? What is the ratio of consumer tax incidence to producer tax incidence? Is this a coincidence? Make a conjecture about what the relationship between the two ratios. Briefly discuss an intuition for this result.
i) [Note: this is a bit of a CHALLENGE QUESTION: don't despair, but do think!] Without fully solving for it , what wou ld be the deadweight loss if we instead used the demand curve from February (i.e. after the demand shift)? (Draw a quick sketch to see what will happen.) What does this tell you about the relationship between deadweight loss and parallel shifts of one of the curves?
Part II – International Trade
Question 2. Suppose Narnia is a small country with a domestic supply and demand for Turkish Delight given by:
Domestic demand : P = 10 – (1/500)Qd
Domestic supply: P = 2 + (1/500)Qs
where P is the price, Qd is the quantity demanded domestically, and Qs is the quantity supplied domestically.
a) Suppose Narnia is currently in autarky (i.e. it does not participate in the international market for Turkish Delight - Narnia's economy is a closed economy when it comes t o Turkish Delight ), what is the market clearing price and quantity in the domestic market? What is Consumer, Producer, and Total Surplus?
b) Narnia now decides to enter the international market for Turkish Delight. Once the market clears, we find that Narnia is importing 2000 units of Turkish Delight. Given this fact, what must the world price? What is the new Consumer, Producer, and Total Surplus? Illustrate your answers graphically.
c) Suppose A slan, ruler of Narnia, fearing that the domestic Turkish Delight industry is unduly suffering from the influx of cheap foreign Turkish Delight, decides to implement a $1 per unit tariff on imports. What is the new price for Turkish Delight in the domestic market, the quantity consumed, the quantity imported, Consumer Surplus, Producer Surplus, Government Revenue, Total Surplus (recalling the convention that we include Government Revenue in Total Surplus), and Deadweight Loss? Illustrate your answers graphic ally.
d) Provide an intuitive explanation for the sources of deadweight loss under this tariff.
e) Suppose, Aslan, seeing the success of this tariff at generating revenue for his government decides to increase the tariff to $3 per unit in the hope of raising e ven more revenue. What is the new quantity consumed domestically, quantity imported, Consumer Surplus, Producer Surplus, Government Revenue, Total Surplus, and Deadweight Loss? Illustrate your answers graphically.
f) Suppose Aslan decides to try an alternative policy. Instead of a tariff, he decides to set an import quota. If Aslan wants Producers to receive as much surplus under this new quota as they do with the $1 tariff, what should he set the quota at? Suppose he decides to auction the import ri ghts to a single firm. At most how much would a firm be willing to pay for the import license?
g) [Note: this is a CHALLENGE QUESTION! You can get there through reasoning or through a mathematical analysis – I would recommend using your comparative advantage when selecting the type of analysis you choose to use.] Aslan quickly realized that increasing the tariff to $3 was a bad idea if he was concerned about revenue; however, he is still interested in finding the tariff that maximizes revenue. Find the reven ue maximizing tariff. Can he do better than the $1 tariff? Provide some intuition for the relationship between revenue and tariff.
Hint: Let T denote the tariff, then use the following facts:
Imports = Qd – Qs
Price = world price + T
0 ≤ T ≤ 2
Think of Imports like a demand curve , but with T instead of P. At what price is revenue maximized on a linear demand curve? Think in terms of elasticity.)
Question 3. Somelandia is a small nation with domestic supply and demand for widgets given by
Domestic Demand: Qd = 300 – 6 P for 0 ≤ P ≤ 50
Domestic Supply: Q s = 2P – 20 for P ≥ 10
where Q is the quantity demanded domestically, Qs is the quantity supplied domestically, and P is the price.
Suppose the World Price for the good is $20.
a) What is the equilibrium price and quantity in autarky (remember "autarky" is the term used to describe a closed market) ?
b) What is the equilibrium price, quantity, imports , consumer and producer surplus if this market is closed to trade? How much is the gain in surplus from international tr ade if this market is opened to trade ? Illustrate your answers graphically. Specifically mark which region represents the surplus gains from trade.
c) Now suppose the government decides to set an import quota of 200 widgets ; i.e. only 200 widgets may be imported. What is the new equilibrium price, quantity, revenue to import license - holders, and deadweight loss?
d) Now suppose the government of Somela ndia decides to try a quota of 80 units. What will be the new equilibrium price and quantity, Consumer and P roducer Surplus, Revenue to license - holders, and Deadweight Loss? Illustrate your answers graphically.
Part III – Elasticities
Question 4. Suppose the demand for gadgets is given by the following equation where P is the price and Q is the quantity demanded: P = 50 – Q
a) Suppose the price is or iginally $10 and increases to $2 0. Using the regular or standard percentage formula, find the price elasticity of demand for gadgets.
b) Suppose the price is or iginally $20 and decreases to $10. Using the regular percentage formula, find the price elasticity of demand for gadgets
c) Now using the arc formula, calculate the pr ice elasticity of demand using the prices from the previous 2 parts. What is the advantage of using the arc formula over the regular percentage formulas?
d) Using the point formula, calculate the price elasticity of demand at a price of $15.
e) Alice is the sole producer of gadgets, and th us gets to set the price. If Alice ’s sole concern is maximizing revenue, what price should s he set?
Question 5. Suppose the demand for frozen chicken nuggets and fres h tuna are given by the following equations where Pn is the price of nuggets (in dollars), Pt is the price of tuna (in dollars), I is median income (in tens of thousands of dollars), and Q is the quantity demanded:
Demand for Frozen Nuggets : Q = 20 – 2Pn – Pt – I
Demand for Fresh Tuna: Q = 40 – 4Pt - Pn + 2I
a) Suppose the price for frozen nuggets is fixed at $2 and the price of tuna is fixed at $4. What is the quantity of frozen nuggets demande d when median income is $10,000? How about when income is $20,000? Given these quantities and using the arc formula, what is the income elasticity of demand for frozen nuggets between these two income levels ?
b) Suppose the prices for nuggets and tuna are still fixed as they were in part (a). What is the quantity of fresh tuna demanded when median income is $10,000? How about $20,000? Given these quantities and using the arc formula, what is the income elasticity of demand for fresh tuna?
c) Given your answers to part s (a) and (b), what can you say about Chicken Nuggets and Fresh Tuna? Are they inferior goods, normal goods and/or luxury goods?
d) Now fix the me dian income at $20,000 and the price of nuggets at $2. If the price of tuna is $4, what is quantity of nuggets demanded? What if the price of tuna is $6? Using this information and the arc formula, calculate the cross - price elasticity of demand for nuggets.
e) Given your answer to part (d), what can you say about the relationship of tuna to nuggets? Are the substitutes or complements ?
Part IV – Consumer Theory
Question 6. Use the following information in answering this next set of questions. Sue likes to eat cheese curds and make long - distance phone calls. Currently, she has no cell phone, and must use a pay phone to make calls, thus she must pay for each call separately at a cost of $5 per call. A dish of Cheese Curds costs $10. Sue’s income is $50.
a) Supposing long - distance phone calls and cheese curds are the only goods Sue buys, plot her budget line with cheese curds on the horizontal axis, and long - distance phone calls on the vertical axis. What is the equation of this line? Use P as the symbol for "phone calls" and C as the symbol for "cheese curds". Label this budget line as BL1 or "budget line part (a)".
b) Suppose Sue’s income increases to $100. Plot her new budget line . What is the equation of this new budget line? In your graph, label this budget line as BL2 or "budget line part (b)
c) Suppose Sue’s income is still $100, but now, due to an unfortunate dairy shortage, the price of cheese curds doubles to $20 a dish. Plot this new budget line. In your graph, label this budget line as BL3 or "budget line part (c)". What is the equation of t he budget line?
d) [Note: (d) and (e) of this problem is a bit of a CHALLENGE and a logic puzzle: do not despair - you can do this, and to get full credit on the homework you CANNOT avoid these challenge questions. So START EARLY AND THINK SERIOUSLY!] Let’s su ppose the dairy shortage has been resolved, so the price of cheese curds is back to normal (i.e. Sue’s budget line is as in part b). A cell phone provider offers Sue a service pl an that costs $50. For the $50, Sue gets 10 long - dis tance calls to start. Afte r the first 10, Sue may pay for additional long - distance calls at a price of $1 per call. Plot the budget line Sue would face if she paid for the service plan. (Hint: First, subtract $50 from Sue’s income to account for the cost of the plan. Be careful; t here will be a kink in the budget line.)
e) Suppose if Sue does not buy the phone service plan, she would consume 8 dishes of cheese curds and 4 long distance phone calls (call this bundle A) . If she purchases the phone plan, she would consume the 5 cheese dishes of cheese curds and use her 10 starter calls, but no more (call this bundle B). Should she purchase the phone plan? Provide some intuition for your solution. (Hint: Sue can afford Bundle A if she doesn’t purchase the service plan since that’s what she consumes , and similarly, she can afford Bundle B if she purchases the service plan. But could Sue have afforded Bundle A with the service plan, and could she afford Bundle B with out the service plan?)
Question 7. Use the following information to answer this next set of questions. Suppose Bob gets Utility from consuming two goods, x and y, according to the following equation where U is the total amount of utility Bob gets measured in utils, X is the amount of g ood X, and Y is the amount of good Y:
U = X*Y
a) Plot indifference curves for Bob for U = 1, U = 2, and U = 3, with X on the x - axis, and Y on the y - axis. Provide equations for each of these curves. What are some properties that make preferences of this sort reasonable in many contexts?
b) Now suppose Bob’s utility takes this form where U is the total amount of utility Bob gets measured in utils, X is the amount of good X, and Y is the amount of good Y:
U = X + Y
Plot indifference curves for U = 1, U = 2, and U =3 , with X on the x - axis, and Y on the y - axis. Provide equations for each of these curves. What is the economic relationship between goods X and Y ?
c) Now su ppose Bob’s utility takes the form where U is the total amount of utility Bob gets measured in utils, X is the amount of good X, and Y is the amount of good Y:
U = min{ X, Y }
i.e . Bob’s level of utility is equal to the amount of the good of which he has less (for example, if (X, Y) = (10, 20) then Bob's level of utility is 10) . Again, plot indiffere nce curves for U = 1, U = 2, and U =3, with X on the x - axis, and Y on the y - axis. What is the economic relationship between goods X and Y ? You do not need to provide equations for these indifference curves.
Question 8. Suppose Alice has preferences for xylophones (x) a nd yachts (y) with utility given by the following equation where U is Alice's level of utility measured in utils, x is number of xylophones and y is number of yachts:
U = x3y
With this utility, Alice has the following marginal utilities:
MUx = 3x2y
MUy = x3
a) Suppose the price of a xylophone is $1,000, and the price of a yacht is $5 ,000. Alice currently has an income of $100,000. What is Alice’s optimal consumption bundle? Remember, the optimal consumption bundle is the point at which Alice equates ma rginal utilities per dollar across both goods and spends all her income. You will have two equations ( one equation equating marginal utilities per dollar and the second equation being the budget constraint) and two unknowns (number of xylophones and number of yachts).
b) Now suppose Alice’s income doubles to $200,000 . What is her new optimal bundle?
c) Now suppose Alice’s income is still $200,000, but the price of yachts doubles to $10,000. Now what is Alice’s optimal bundle?
d) Draw a well labeled graph that illustrates the income and substitution effects of this price change described in (c) . [Note that you will not have the numeric values for (x, y) for point C: just indicate where "C" should be given your analysis.]
e) Notice this increase in price has reduced Alice’ s total consumption, so her welfare is negatively affected by the change. How much extra income would you have to give Alice to compensate her for this change? That is, how much additional income would she need so that her optimal bundle with the new prices would lie on her previous indifference curve? (You will need a calculator for this part .)
Part V – Real vs Nominal (You may need a calculator for certain parts of the next 2 questions.)
Question 9. Suppose prices for goods in some fictional Midwestern city over time are given by the following table:
Year Price of Cheese Curds Price of a Sweater Price of a Gold Bar
2010 $5 $25 $500
2011 $6 $25 $800
2012 $6 $30 $900
a) Suppose the average market bask et in this city is composed of 3 00 dishes of Cheese Curds, 50 Sweaters, and 1 Gold Bar. Calculate the cost of the market basket in each year.
Year Cost of Market Basket
2010
2011
2012
b) Now, using 2010 as the base year, calculate the CPI for each year using a 100 point scale . Then, for 2011 and 2012, calculate the annual inflation rate. Show your answers to two decimal places.
Year CPI Inflation rate
2010 -
2011
2012
c) Now, still using 2010 as base year, calculate the real price of cheese curds in each year. Again, show your answers to two decimal places.
Year Real price of cheese curds
2010
2011
2012
Question 10. Now, using everything you’ve learned so far about real and nominal prices, fill in the blanks of the following table. The market basket for this economy is composed of 10 loaves of bread and 5 gallons of milk. Compute real prices using a base year of 2005 and a CPI computed on a 100 point scale . Express your answers to 2 decimal places when necessary