Examines the information about particular stocks

Question

Discuss the below:

Q: David wants to invest his $4 million inheritance. He walks into Stock-o-rama and examines the information about four particular stocks. David will sell his stocks after exactly one year.

Each of the stocks will pay out a particular amount in one year, times the amount invested. According to the experts at Stock-o-rama, the following vectors represent the mean return per dollar invested for each stock, and the standard deviations of each stock's return.

	Stock 1	Stock 2	Stock 3	Stock 4
Mean	1.04	1.09	1.03	1.07
Standard Deviation	0.03	0.04	0.01	0.05

So, if David invests $1 million in Stock 1, then the mean amount of money he will get in one year is $1.04 million, and the standard deviation of the amount of money is $0.03 million.

The Stock-o-rama experts also claim that the returns of the four stocks are relevant to each other. The following matrix represents the correlation of each stock with each other.

	Stock 1	Stock 2	Stock 3	Stock 4
Stock 1	1	-0.06	0.44	0.71
Stock 2	-0.06	1	0.09	0.60
Stock 3	0.44	0.09	1	-0.81
Stock 4	0.71	0.60	-0.81	1

David will invest his $4 million in these four stocks, and cares about the amount of money he will get in one year. His portfolio will be worth the sum of the worth of the stocks he buys.

He likes making a lot of money, but doesn't like the risk of a high variance. He decides to choose his portfolio based on the following formula. If x‾ represents the mean amount of money his portfolio will be worth in one year, and

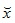represents the variance of that amount of money, then David wants to maximize where t = $5 million.(Here, t is a measure of David's fear of high variance, known as David's risk tolerance)

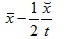
Which of the following portfolios will David prefer?

a) $4 million invested in Stock 2

b) $2 million each in Stock 2 and Stock 4

c) $2 million each in Stock 3 and Stock 4

d) $1 million in each stock

And calculate the mean and variance of each of the four portfolios a, b, c, and d.