Examine the number of maryland households

Question

Assignment:

Question 1. A person's gender and the choice of their pet was recorded when 550 people were surveyed.

	Dog	Cat	Other Pet	No Pet
Female	120	132	18	30
Male	135	70	20	25

What is the probability a person owns a cat given they are a female?

• 0.24
• 0.44
• 0.367
• 0.653

Question 2. A person's gender and the choice of their pet was recorded when 550 people were surveyed.

	Dog	Cat	Other Pet	No Pet
Female	120	132	18	30
Male	135	70	20	25

What is the probability a person is male given they own a dog?

• 0.54
• 0.464
• 0.245
• 0.529

Question 3. The table below shows drivers on New Year's Eve. It outlines whether they are driving intoxicated and whether an accident occurred. If a driver is chosen randomly what is the probability they had an accident given they were driving intoxicated? Round your answer to 2 decimal places.

	Driving Intoxicated	Not Driving Intoxicated	Total
Accident	9	5	14
No Accident	39	97	136
Total	48	102	150

Question 4. A researcher wants to examine the number of Maryland households that will buy a house within the next year. If three Maryland households are selected randomly, let X be the number of the households that intend to purchase a house within the next year. What are the values that the random variable can take on?

• 1, 2, 3
• 0, 1, 2
• 0, 1, 2, 3

Question 5. A doctor at a small rural hospital wants to research the number of babies born daily at their hospital. The maximum number ever born in one day is 6 babies. Let Y be the number of babies born at this hospital in a day. What are the values that the random variable can take on?

• 0, 1, 2, 3, 4, 5, 6
• 1, 2, 3, 4, 5, 6
• 0
• 6

Question 6. One of the requirements of a probability distribution is that the probabilities must sum to 0.

• True
• False

Question 7. The probability model below describes the number of thunderstorms that a certain town may experience during the month of August. What should the value be in the missing cell?

X	P(x)
0	0.08
1	0.25
2	0.33
3	0.18
4
5	0.07

• 0.17
• 0.09
• 0.20
• 0.90

Question 8. Sue Anne owns a medium-sized business. The probability model below describes the number of employees that may call in sick on any given day. What is the probability that 1 employees calls in sick?

X Number of Employees Sick	P(x)
0	0.05
1	0.40
2	0.30
3	0.20
4	0.05

Question 9. Sue Anne owns a medium-sized business. The probability model below describes the number of employees that may call in sick on any given day. What is the probability that more than 2 employees call in sick?

X Number of Employees Sick	P(x)
0	0.05
1	0.40
2	0.30
3	0.20
4	0.05

Question 10. The following probability model describes the number of golf balls ordered by customers of a pro shop and the corresponding probabilities. What is the mean number of golf balls ordered?

X	P(x)
3	0.14
6	0.29
9	0.36
12	0.11
15	0.10

• 5
• 8.22
• 9
• 0.20

Question 11. Del Taco (a restaurant) managers recorded the number of street tacos, X, ordered by tables dining. They observed that X had the following probability distribution. What is the expected number of street tacos that a table will order?

X(Number of Street Tacos)	P(x)
0	0.28
1	0.33
2	0.19
3	0.13
4	0.05
5	0.02

Question 12. A game: You roll two dice. If the number of dots showing are greater than 7, you win $2. If the number of dots showing are 7 or less, you lose $1. Which model below correctly outlines the probability distribution for this game.

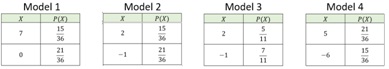
• Model 1
• Model 2
• Model 3
• Model 4

Question 13. A game: You roll two dice. If the number of dots showing are greater than 7, you win $2. If the number of dots showing are 7 or less, you lose $1. What is the expected value of this game?

M2:L5

Question 14. Which of the following is NOT an assumption of the Binomial distribution?

• The probability of success is equal to 0.5 in all trials.
• There are a fixed number of trials.
• There are two possible outcomes (success and failure).
• The probability of a success, p, is constant.

Question 15. Is the binomial distribution appropriate for the following situation:

Joe buys a ticket in his state's "Pick 3" lottery game every week; X is the number of times in a year that he wins a prize.

• Yes
• No

Cannot determine from information given

Question 16. Is the binomial distribution appropriate for the following situation:

Consider the experiment where four marbles are drawn without replacement from an urn containing 35 yellow and 65 blue marbles, and the number of yellow marbles drawn is recorded.

• Yes
• No

Question 17. Suppose a manufacturer of transistors believes that, on the average, 1 defective transistor occurs in every 100 transistors. A batch of 25 transistors is randomly selected.

Let a "success" be the transistor is defective.

Identify the following:

n =
p =

Question 18. Five percent of the eyeglasses sold at an optical retailer have tinted lenses. Forty pairs of glasses are sold on a particular day and six have tinted lenses. Identify n, p, and x.

n =
p =
x =

Question 19. Only 35% of the drivers in a particular city wear seat belts. Suppose that 20 drivers are stopped at random what is the probability that exactly four are wearing a seatbelt? (Round your answer to 4 decimal places)

Question 20. Write a statement explaining what the probability means in the previous problem.

Question 21. In a certain college, 33% of the physics majors belong to ethnic minorities. If 8 students are selected at random from the physics majors, what is the probability that more than 5 belong to an ethnic minority?

• 0.0659
• 0.9154
• 0.0846
• 0.0187

Question 22. A laboratory worker finds that 3% of his blood samples test positive for the HIV virus. In a random sample of 70 blood tests, what is the mean number of people that test positive for the HIV virus? (Round your answer to 1 decimal place)

Question 23. A laboratory worker finds that 3% of his blood samples test positive for the HIV virus. In a random sample of 70 blood tests, what is the standard deviation of the number of people that test positive for the HIV virus? (Round your answer to 1 decimal place)

Question 24. Using the information from the two previous problems, would it be unusual if 6 blood samples tested positive for HIV?

• No
• Yes