Task:
Energy Economics
1. Suppose the government wishes to regulate mercury emissions of factories in a specific industry by either setting an emissions standard or imposing an emissions fee (per tonof mercury). The government is uncertain as to the marginal abatement costs, which
may be high (MC1) or low (MC2).
MC1 = 15M + 500
MC2 = 15M − 500
where M is the units of mercury abated. The government believes there is a 50% chance of each of the marginal abatement costs. The marginal benefit of abatement is known to be: MB = 1500 − 10M
(a) What is the optimal level of emissions for each of the cost curves above?
(b) What is the expected marginal abatement cost (equation)?
(c) What is the optimal emissions standard according to the expected abatement costs?
(d) What is the optimal abatement fee according to the expected abatement costs?
(e) Which regulation will result in a lower DWL in the presence of the uncertainty?
Explicitly compute the potential DWL arising from each proposal.
2. Suppose that the market for steel is shown in figure 2.
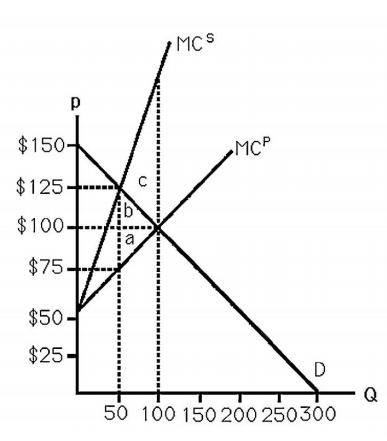
(a) What is DWL (including externalities) under perfect competition and no environmental regulation? (Calculate the amount)
4 Problem Set 1
(b) What is DWL assuming this market is a monopoly (and no environmental regulation)? (Calculate the amount)
(c) What would be the optimal pollution tax if it has to be a fixed dollar amount assuming the market is perfectly competitive?
3. Assume that there are two power plants (firm 1 and firm 2) emitting sulfur dioxide, an air pollutant. They can reduce emissions at the following marginal costs, where q1 is tons of emissions abatement by firm 1, and q2 is tons of abatement by firm 2:
MC1 = 8q1; MC2 = 2q2
Assume that unregulated emissions are 40 tons for firm 1 and 20 for firm 2, for a total of 60 tons and that fixed costs are zero.
(a) An environmental regulator must reduce total emissions of sulfur dioxide by 20 tons. Compute the cost-effective allocation of control responsibility; how many tons of emissions abatement must each firm achieve in order to minimize the total abatement costs?
(b) If the regulator chooses to meet her 20-ton reduction objective with an emissions tax, what per-unit charge should be imposed? How much government revenue will the tax system generate, if the tax is levied on all units of pollution?
(c) The aggregate marginal cost function for the two-firm industry is MC(Q) = 1.6Q where Q is total abatement. (It would be good practice to verify this graphically or algebraically.) Assume the marginal benefit function for sulfur dioxide pollution abatement is
MB = 50 − 0.4Q
What is the efficient level of pollution control (call it Q*)? Is the tax you calculated in part (b) just right, too low, or too high to induce the efficient level of abatement? What emission tax would achieve the efficient level of control?
(d) Now, if firms (and the regulator) are uncertain regarding the costs of pollution control, so that the aggregate marginal cost function, given previously, represents expected marginal costs, would you argue that a pollution tax or an equivalent tradable permit system would be more efficient? Please use a graph to explain your reasoning.
4. Since the city of Davis thinks “light pollution” is bad, neighborhoods have to fund their own streetlights. Suppose three neighbors must vote on the installation of a light that costs $210.
The cost of the light will be shared by all three. Al values the light at $50; Belinda values the light at $50; and Chaz (who rides their bike at night) values the light at $200. If the voting rule is that the majority wins, does the light get purchased? Is it efficient to purchase the light?