Why?
When you buy a bottle of a certain brand of lemonade you expect it to taste just as sweet as the last time you bought that kind of lemonade. Likewise, when doctors prescribe a certain ointment, they expect the concentration of medicine to be consistent. How do companies ensure their products taste or perform the same every time you purchase them? Many companies, including pharmaceutical companies, keep track of the concentration of a solution by measuring its molarity—a ratio of number of solute particles to the volume of solution. In this activity you will learn about molarity and how to represent concentration quantitatively.
Model 1 – Lemonade Mixtures
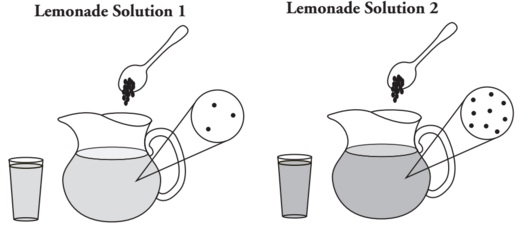
*Both pitchers were filled with enough water (solvent) to provide 2 liters of solution.
Dissolved Lemonade Mix particle (solute) = •
1. Refer to Model above.
a. What is the solvent in this scenario?
b. What is the solute in this scenario?
c. What is a dissolved lemonade mix particle represented by?
2. Circle the word that best completes each sentence below and justify your answer based on the diagrams in Model above.
a. Lemonade Solution 1 has (more/less/the same) volume of solution as 2. How do you know?
b. Lemonade Solution 1 has (more/less/the same) quantity of solute as Solution 2. Describe how you know in terms of number of particles.
3. Lemonade Solution 2 is considered to be concentrated, and lemonade Solution 1 is considered to be dilute. Examine the two pictures in Model 1. List two ways to differentiate a concentrated solution from a dilute solution.
4. A glass is filled with the concentrated lemonade solution from Model above.
a. Is the solution in the glass the same concentration as the solution in the pitcher?
b. Does the solution in the glass contain the same number of solute particles as the solution in the pitcher? If no, explain how your answer to part a can be true. Hint: Consider both amount of solute and amount of solvent.
5. Do the terms “concentrated” and “dilute” provide any specific information about the quantities of solute or solvent in a solution? Explain.
Model 2 – Chemical Solutions:

6. List the beaker numbers for the solutions in Model 2 that are considered to be “concentrated.”
7. What does the letter “M” stand for in Model 2?
8. Use the data in Model 2 to answer the questions below.
a. Calculate the ratio of the moles of solute to liters of solution for each solution.
moles of solute/liters of solution
b. How does the ratio compare to the molarity of each solution?
9. Write a mathematical equation to show how the molarity of a solution is calculated.
10. Which type of solution (dilute or concentrated) will have a larger molarity value?
11. Consider beakers 3–5 in Model 2. Circle the answer below for the quantity that is the same in all of the beakers that contain 3 M solutions.
Number of moles of solute
Volume of solution
Ratio of moles of solute to liters of solution
12. Explain how beaker 5, with fewer moles of glucose, can have the same molarity as beaker 4.
13. Can molarity be determined by knowing either the number of moles alone or the volume of solution alone? Explain why or why not.
14. Calculate the molarity of a solution containing 1.5 moles of NaCl in 0.50 liters of solution. Show your work.
15. Calculate the molarity of a solution containing 0.40 moles of acetic acid in 0.250 liters of solution. Show your work.
16. A 0.5 M KCl solution contains 74.55 g of KCl (molar mass 74.55 g/mol) in 2000 mL of solution.
a. Does the ratio 74.55 g KCl/2000 ml provide the correct molarity for this solution?
b. What units do the amount of solute and the volume of solution need to be in to obtain the molarity of 0.5 M? Show the calculation.
17. Calculate the molarity of a solution containing 2.5 g of CuCl2 in 1 L of solution. Show your work, including units.
18. Consider the 3 M solutions in Model 2. What would a 3 M HCl picture look like if the beaker contained 100 mL? In the circle below, visually show the number of solute particles that would be present in any very small volume of the overall solution. (See Model for examples.)
-
19. A student thinks that she can determine the concentration of a solution based on the darkness or color intensity of the solution alone. Based on the pictures in Model 2 describe why this student’s method will not always work.
20. Calculate the molarity of a KCl solution if 37.3 g of KCl are dissolved in water to give a final solution volume of 500 mL. Show your work.
21. A student correctly determines that 17.1 grams of sucrose are needed to make 50 mL of a 1 M sucrose solution. When making this solution in lab, the student measured 50 mL of water and massed 17.1 grams of sucrose. The student then mixed the two together into a new beaker. The student’s resulting solution was not 1 M sucrose as intended. After the student mixed sucrose and water, the resulting solution was poured into a graduated cylinder and it read 62 mL.
a. What part of the molarity equation did the student overlook when mixing the solution?
b. What steps should the student take in lab to correctly prepare the 1 M solution?