Determining the solution of the homogeneous system

Question

Question1 The reduced row echelon form of

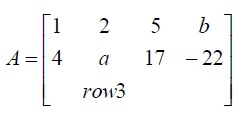is equal to

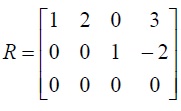
(a) What can you say about row 3 of A? Give an example of a possible third row for A.

(b) Determine the values of a and b.

(c) Determine the solution of the homogeneous system of equations Rx = 0 in parametric vector form.

(d) What is the dimension of the column space of A? Do the columns of A span R³ ?

Question 2 Suppose an economy has four sectors, Agriculture (A), Energy (E), Manufacturing (M), and Transportation (T). Sector A sells 10% of its output to E and 25% to M and retains the rest. Sector E sells 30% of its output to A, 35% to M, and 25% to T and retains the rest. Sector M sells 30% of its output to A, 15% to E, and 40% to T and retains the rest. Sector T sells 20% of its output to A, 10% to E, and 30% to M and retains the rest.

(a) Construct a diagram that shows the exchange between the three sectors.

(b) Determine the exchange table for this economy, where the columns describe how the output of each sector is exchanged among the four sectors.

(c) Denote the prices of the total annual outputs of the sectors by pA, pE, pM and pT respectively. Determine the equations that need to hold for the equilibrium prices for the four sectors.

(d) Find the equilibrium prices, if they exist.

Question 3

Balance the following chemical equation using the vector equation approach. PbN₆ + CrMn₂O₈ → Pb₃O₄ + Cr₂O₃ + MnO₂ + NO

Question 4

Let

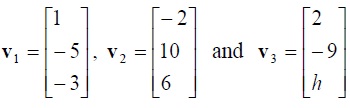
(a) For what values of h is 3 v in Span {v₁, v₂}? Justify your answer.

(b) For what values of h is {v₁, v₂, v₃} linearly dependent? Justify your answer.

Question 5
Consider the linear transformation (a) Find the image of (3, - 2, 2) under T.

(b) Does the vector (5, 3) belong to the range of T?

(c) Determine the matrix of the transformation.

(d) Is the transformation T onto? Justify your answer

(e) Is the transformation one-to one? Justify your answer

Question 6

a) For each of the following matrices explain why the matrix is not invertible.
i)

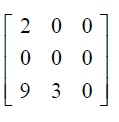
ii)

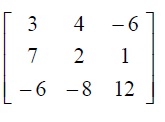
iii)

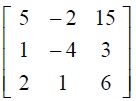
b) Suppose A is an n×n matrix with the property that the equation Ax = 0 has only the trivial
solution. Without using the Invertible Matrix Theorem, explain directly why the equation
Ax = b must have a solution for each b in Rn