Questions:
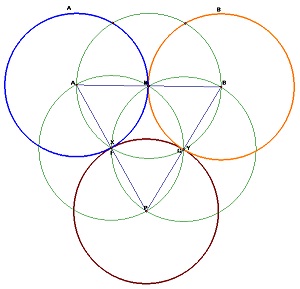
Tangency of three circles
1. Create two tangent circles with centers A and B. For convenience sake, let circle A have the larger radius.
2. Find the midpoint of segment AB. Call it M.
3. Draw the circle with center M passing through A and B. Label this circle's lower intersection point with circle A as F and that with circle B as G.
4. Draw the circle whose center is F that passes through G and the circle whose center is G that passes through F -- the classic Euclidean construction of an equilateral triangle.
5. Label the lower intersection point of circles F and G as P. Connect P to A and label the intersection of segment PA and circle A as X. Connect P to B and label the intersection of segment PB and circle B as Y.
The circle whose center is P that passes through X and Y appears to be tangent to the original circles A and B. Please prove why this is